Capital Structure
After completing this reading, you should be able to: Explain different methods to raise capital. Understand the two main forms of financing: equity issues and debt issues. Understand the process by which a company raises capital including venture capital, IPOs,…
Investment Risk and Project Analysis
After completing this reading, you should be able to: Discuss the advantages and disadvantages of different measures of investment risk. Understand the properties, advantages, and disadvantages of the various measures of investment risk: Variance, Semi Variance, Value at Risk (VaR),…
Market Efficiency and Behavioral Finance
After completing this reading, you should be able to: Explain the three forms of Market Efficiency (EMH) Understand the definition of efficient markets, and distinguish between the strong, semi-strong and weak versions of the EMH. Identify empirical evidence for or…
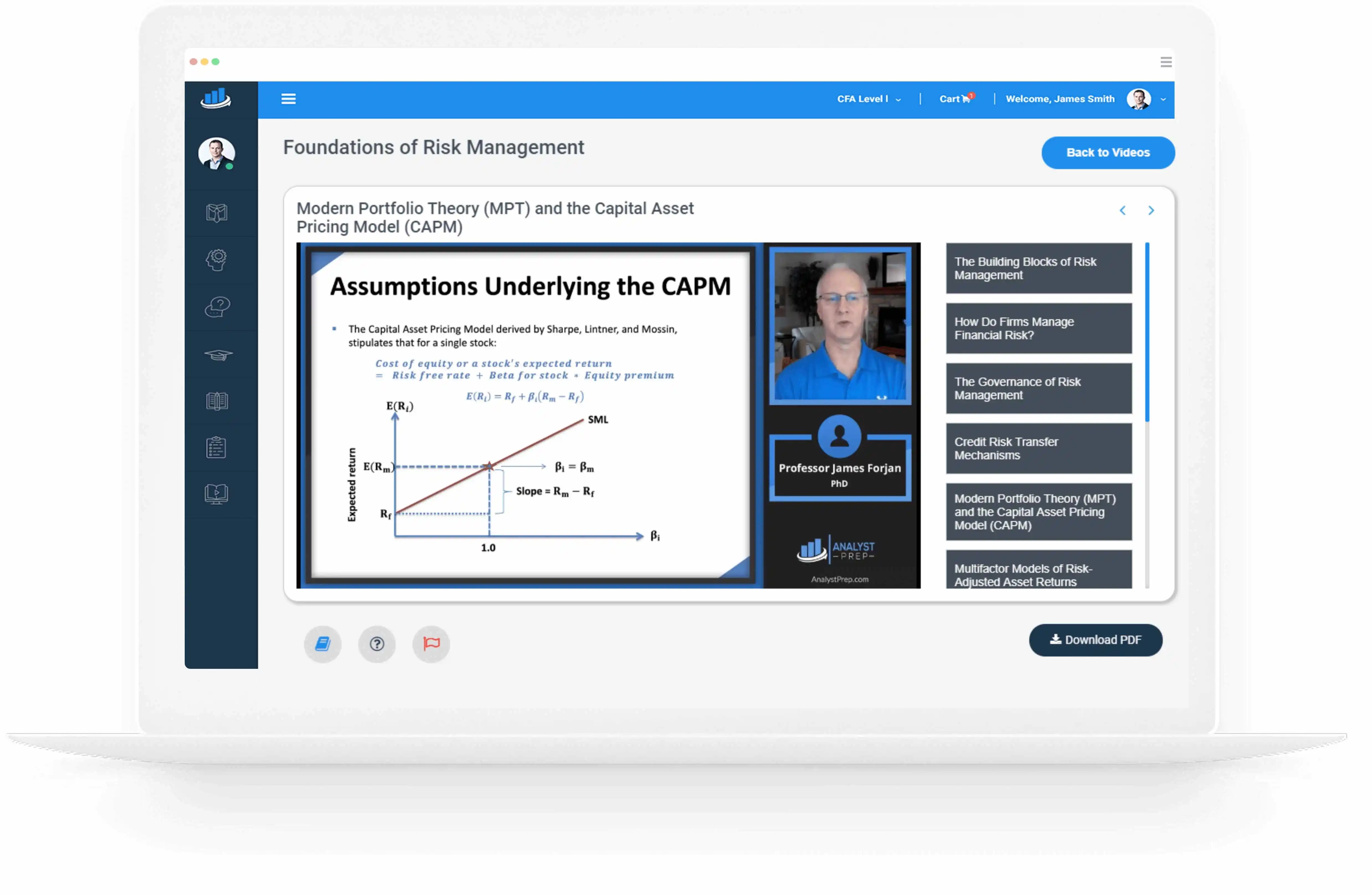
Asset Pricing Models
After completing this chapter, the Candidate will be able to: Explain the Capital Asset Pricing Model (CAPM). Recognize the assumptions and properties of CAPM Calculate the required return on a particular asset, a portfolio or a project using CAPM. Explain…
Mean-Variance Portfolio Theory
After completing this reading, you should be able to: Explain the mathematics and summary statistics of portfolios. Calculate the risk and return of an asset, given appropriate inputs. Calculate the risk and expected return of a portfolio of many risky…
Exam P Syllabus – Learning Outcomes
General Probability 1.a – Define set functions, Venn diagrams, sample space, and events. Define probability as a set function on a collection of events and state the basic axioms of probability. 1.b – Calculate probabilities using addition and multiplication rules. 1.c – Define…
State and apply the Central Limit Theorem
For this learning objective, a certain knowledge of the normal distribution and knowing how to use the Z-table is assumed. The central limit theorem is of the most important results in the probability theory. It states that the sum of…
Calculate probabilities for linear combinations of independent normal random variables
Definition: Let \(X_1, X_2,\ldots,X_n\) be random variables and let \(c_1, c_2,\ldots, c_n\) be constants. Then, $$ Y=c_1X_1+c_2X_2+\ldots+c_nX_n $$ is a linear combination of \(X_1, X_2,\ldots, X_n\). In this reading, however, we will only base our discussion on the linear combinations…
Determine the distribution of a transformation of jointly distributed random variables
Transformation for Bivariate Discrete Random Variables Let \(X_1\) and \(X_2\) be a discrete random variables with joint probability mass function \(f_{X_1,X_2}(x_1,x_2)\) defined on a two dimensional set \(A\). Define the following functions: $$ y_1 =g_1 (x_1, x_2)$$ and $$y_2 =g_2(x_1,x_2)$$…
Calculate joint moments, such as the covariance and the correlation coefficient
Let \(X\) and \(Y\) be two discrete random variables, with a joint probability mass function, \(f\left(x, y\right)\). Then, the random variables \(X\) and \(Y\) are said to be independent if and only if, $$ f\left(x,\ y\right)=f\left(x\right)\times f\left(y\right),\ \ \ \…