Analysis of Variance (ANOVA)
The sum of squares of a regression model is usually represented in the Analysis of Variance (ANOVA) table. The ANOVA table contains the sum of squares (SST, SSE, and SSR), the degrees of freedom, the mean squares (MSR and MSE),…
Coefficient of Determination and F-statistic
Sum of Squares Total (SST) and Its Components Sum of Squares Total (total variation) is a measure of the total variation of the dependent variable. It is the sum of the squared differences of the actual y-value and mean of…
Assumptions Underlying Linear Regression
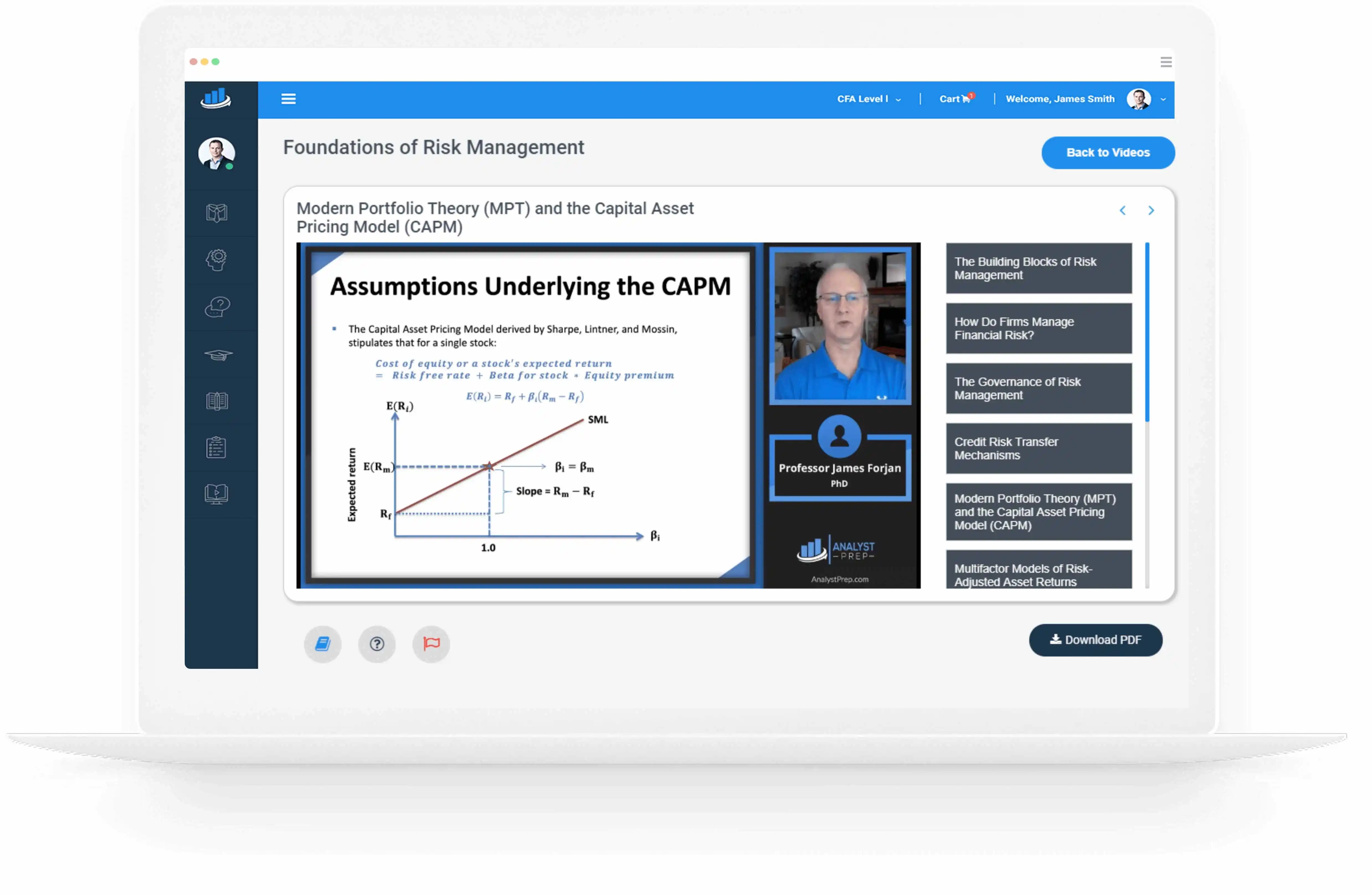
The classic normal linear regression model assumptions are as follows: I. The relationship between the dependent variable, Y, and the independent variable, X, is linear. A linear relationship implies that the change in Y due to a one unit change…
The Least Squares Criterion
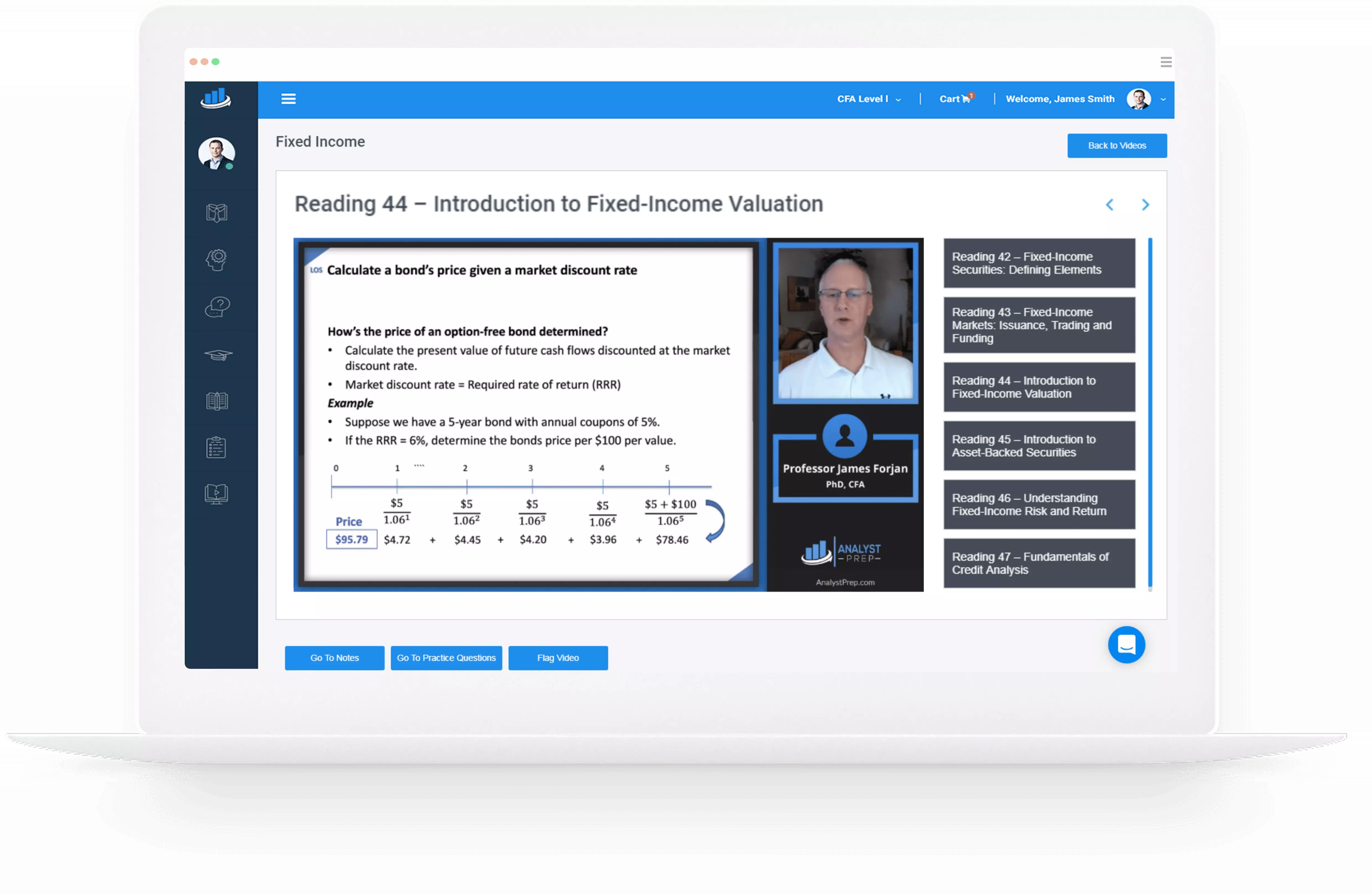
The linear relation between the dependent and independent variables is described as follows: $$Y_i =\beta_0+\beta_1X_i+\epsilon_i,\ i=1,2,…,n$$ Where: \(Y\) = dependent variable. \(X\) = independent variable. \(\beta_0\) = intercept. \(\beta_1\) = slope coefficient. \(\epsilon\) = error term which is the observed…
Dependent and Independent Variables
Linear regression forecasts the value of a dependent variable given the value of an independent variable. It assumes that there is a linear relationship between dependent and independent variables. A dependent variable is predicted by an independent variable and is…
Analytical Duration and Empirical Duration
[vsw id=”rRcQKHgC16c” source=”youtube” width=”611″ height=”344″ autoplay=”no”] Differences between Analytical Duration and Empirical Duration Analytical duration refers to estimating duration and convexity using mathematical formulas (as done in the previous learning objectives). Analytical duration approximates the effect of changes in benchmark…
Compare Nominal and Real GDP and Calculate and Interpret the GDP Deflator
[vsw id=”cRMasYNNsVA” source=”youtube” width=”611″ height=”344″ autoplay=”no”] It is economically healthy to exclude the effect of general price changes when calculating the GDP. This is because higher (lower) income caused by inflation does not indicate a higher (lower) level of economic…
Short-run Macroeconomic Equilibrium Above or Below Full Employment
[vsw id=”cRMasYNNsVA” source=”youtube” width=”611″ height=”344″ autoplay=”no”] Short-run macroeconomic equilibrium only occurs when the amount of real GDP demand equals the amount of GDP supply. On a graph, this happens at the point where the AD curve intersects the short-run average…
Functions and Definitions of Money
[vsw id=”KKHi1HKTpD4″ source=”youtube” width=”611″ height=”344″ autoplay=”no”] Definitions of Money According to Growther, money refers to anything that is generally accepted as a means of exchange. What’s more, it is that which at the same time acts as a measure and…