Regression with Multiple Explanatory V ...
After completing this reading, you should be able to: Distinguish between the relative... Read More
After completing this reading, you should be able to:
A short hedge occurs when the trader shorts (sells) a futures contract to hedge against a price decrease in an existing long position. A short hedger already owns the underlying asset or is likely to gain ownership of the asset in the near future, after which they will sell it. When the hedged/underlying asset price decreases, the short futures position realizes a corresponding positive return that offsets the loss.
Therefore, a short hedge is suitable when:
Consider the following example.
Example: Calculating the Profit or Loss on Short Position
Oelig is an oil refining company and a member of the CME Group. The company will receive 5 million barrels of oil in two months. The company will sell the oil as soon as it receives it. The company knows that due to the large amount of oil it expects to receive, it will lose (gain) a whopping $50,000 if the price per barrel decreases (increases) by just one cent (i.e., 5,000,000 × 0.01 = 50,000). Although there’s a chance of a gain, the company feels this is a risk too big to take.
To hedge the risk of loss, the company enters into a short position. Assume that each traded futures contract involves the purchase or sale of 1,000 barrels of oil. Further, let’s assume that Oelig receives the oil during the delivery period of the contract.
Suppose that the current spot price per barrel $60, and the three-month futures price is 60.50. Now consider the following scenarios:
Scenario 1: At the time of delivery, the spot price per barrel of oil is $55
Scenario 2: At the time of delivery, the spot price per barrel of oil is $65
Let’s now see what each of the above scenarios would mean for Oelig:
Scenario 1
Assuming the company did not enter the futures contract, the price received for the oil in the market would be $275m: $$5,000,000\times 55 =\$275,000,000$$ In reality, the company is obliged to deliver under the futures contract. The profit made amounts to $27.5m: $$ 5,000(60.5-55)\times1,000=\$ 27,500,000$$
If we combine the price received for the oil in the market with the gain on the 5,000 futures contracts, the net price received is $302.5m: $$$275,000,000+27,500,000=$302,500,000$$
This translates to a net price of $ 60.50 per barrel (=$305,500,000/5,000,000). A closer look reveals that this matches the price locked in the futures contracts.
Scenario 2
Assuming the company did not enter the futures contract, the price received from the oil in the market would be $325m: $$5,000,000\times65=\$325,000,000$$
Under the futures contract, however, the company would be obliged to sell at the futures price of $60.5 per barrel. In these circumstances, the company would make a loss amounting to $22.5m: $$5,000\left(60.5-65\right)\times1,000=$22,500,000$$
When this loss is taken into account, the net price received for the oil is again $302.5m:
$$\$325,000,000-22,500,000=$302,500,000$$
Thus, the net price per barrel is $60.50 (=$302,500,000/5,000,000).
What this example shows, therefore, is that a combination of the market price of an asset at the maturity of a futures contract and the gain/loss on the futures contract should always work out to the futures contract price. It suffices to say that the hedge works as intended when the price of the asset declines between the time the contract is initiated and its maturity. If it increases, the hedger actually makes a loss.
A long hedge occurs when the trader buys a futures contract to hedge against a price increase in an existing short position. A long hedger plans to buy the underlying asset in the future and fears a price rise, triggering a loss. When the hedged/underlying asset price increases, causing a loss, the long futures position realizes a corresponding positive return that offsets the loss in asset value.
Basis risk is the risk that the value of a futures contract will not move in a normal, steady correlation with the underlying asset price. For example, if the current spot price of gold is $1,500, and the six-month futures price of gold is $1,550, then the basis is $50. Basis risk, in this case, is the risk that between now and the maturity of the contract in six months, the price of gold will fluctuate by more than $50.
A trader is exposed to basis risk if they close out a futures contract before its maturity. The basis is the difference between the spot price and the futures price, and the basis risk the risk associated with the basis at the time of closing out a contract.
$$ \text{Basis}=\text{Spot Price}-\text{Futures Price} $$
If the asset being hedged is different from the underlying asset in the futures contract, Then;
$$ \text{Basis}=\text{Spot Price of the hedged asset}-\text{Future price of the underlying asset in the futures contract} $$
The fluctuation in the basis makes hedges less effective than they are meant to be. Between contract initiation and liquidation, the price spread (the difference between the cash and futures price) may narrow or widen.
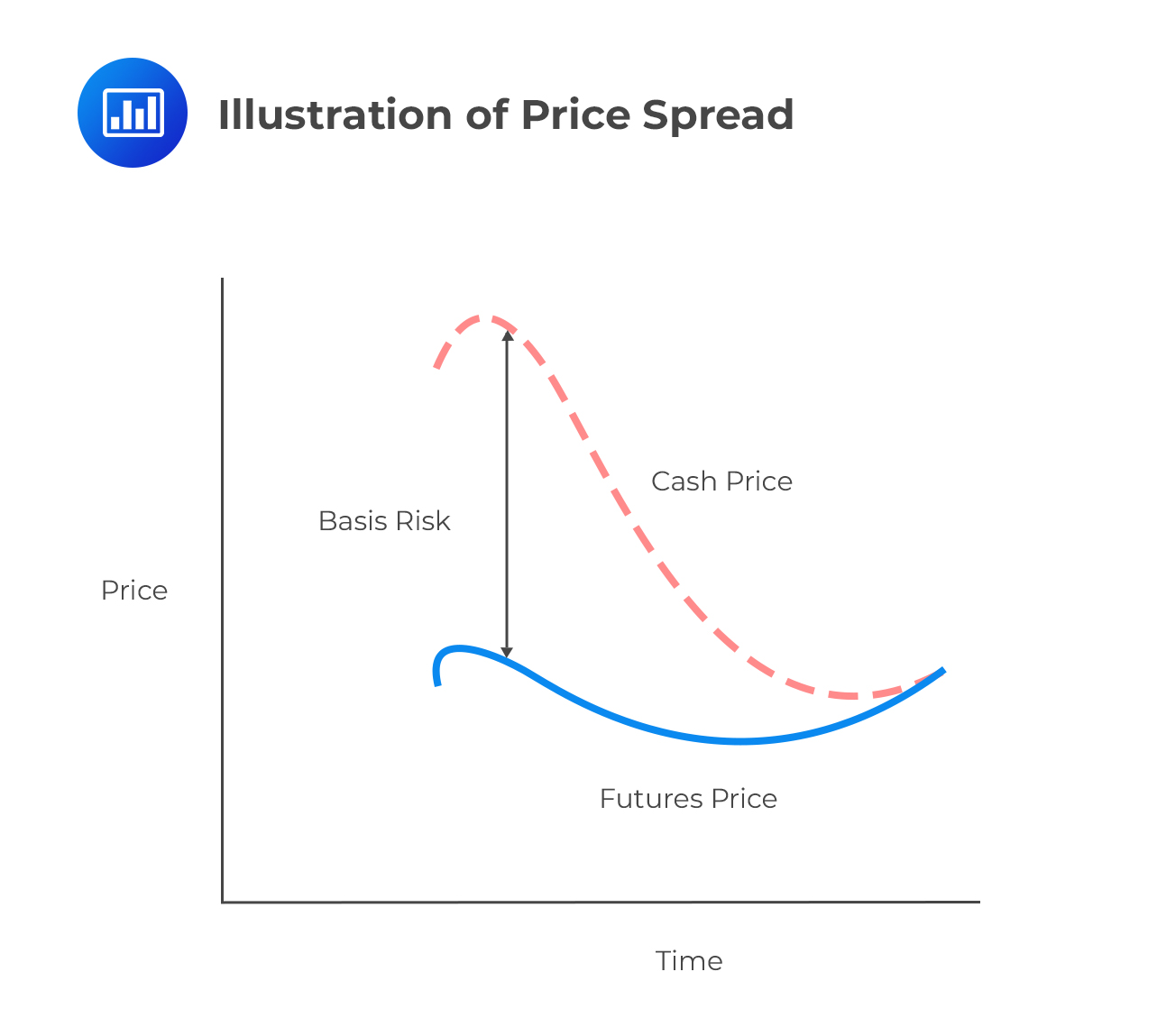 Sources of Basis Risk:
Sources of Basis Risk:To minimize basis risk, choosing the hedging tool that’s most correlated with the underlying is imperative.
Consider an investor who is due to sell an asset at time \(t\) (future), and thus, the investor enters into a short position.
Define the following terms:
\(F_0\) = Future Price at the time of initiation of a contract.
\(F_t\) = Future Price at the time the contract is closed out.
\(S_t\) = Spot price at the time the contract is closed out.
\(b_t\) = Difference difference between the spot price and the futures price at time t (Basis at time \(t = S_t-F_t\))
At time \(t\) the investor in short position will receive a price of \(S_t\) from the asset sold and the profit from the short position in a futures contract will be \(F_0-F_t\). Thus,
$$\begin{align}\text{Net Price Received from a Short Hedge} &= S_t +(F_0-F_t)\\ &=F_0+(S_t-F_t)\\ &=F_0+b_t \end{align}$$
From the expression above, it is easy to see that if the spot price is the same as the futures price when the hedge closed out, then the basis is zero, and thus the net price received is equivalent to the futures price (\((F_0)\). On the flip side, if the hedge is closed out before the delivery period and the underlying asset is different from the one delivered, the hedger is subject to basis risk.
Example: Short Hedge and Basis Risk
A farmer will need to sell 200,000 bushels of corn in January. To hedge his position, the farmer uses February CME Group’s futures contracts, which are set such that each contract is on 10,000 bushels of corn. At the hedge initiation, the futures price is $3.50 per bushel, and when the hedge is closed out, the futures price was $3.75 per bushel in January.
The spot price for corn sold in January is $3.80 per bushel. What is the net price received per bushel if the farmer closes out his hedge in January?
Solution
If the farmer closes out his position in January, the net price received from the corn is given by: $$200,000\times3.80+20\times10,000\times\left(3.50-3.75\right)=\$710,000$$
Thus, the net price received by the farmer is: $$\frac{710,000}{200,000}=$3.55\ per\ bushel$$ The result above can be explained as follows: The price of corn increases, so the farmer loses $0.25 (=$3.75-$3.50) per bushel when he closes his futures contracts. Instead, there is a basis of $0.05 ($3.80-$3.75) per bushel, which implies that when the farmer sells the corn at a price of $3.80 per bushel, the basis ($0.05) and the loss ($0.25) combine to reduce the price received per bushel to $3.55 per bushel.
Consider an investor who will buy an asset in the future, say at time \(t\). At time \(t\), the investor will pay a price of \(S_t\) for the asset received, and the profit on the long position in a futures contract is \(F_t-F_0\). Therefore,
$$\begin{align} \text{Net Cost of an asset on long hedge} &= S_t-(F_t-F_0)\\ &=F_0 +(S_t-F_t)\\ &=F_0+b_t \end{align}$$
From the above equation, the net price received for the short hedge and the long hedge is both equal to \(F_0+b_t\).
In the context of hedging and basis risk, futures price (F_0) is known when the hedge is initiated; however, the outcomes of hedging depend on uncertainty of the futures’ basis (b_t).
Example: Long Hedge and Basis Risk
A US-based company will need to buy GBP 437,500 in March. The company wishes to hedge itself against the exchange rate risk using the CME Group’s April futures contract. CME Group trades GBP 62,500 per contract.
At the hedge initiation, the futures price was USD 1.30 per GBP, and at the time of the hedge close out (April), the futures price was USD 1.32 per GBP. However, the spot price in April is USD 1.35 per GBP.
What is the net exchange rate for the company when the hedge is closed out?
Solution
The company needs 7 (=437,500/62,500) CME Group’s futures contract to hedge its position. As such, the value of British pounds is given by: $$437,500\times1.35-\left[\left(1.32-1.30\right)\right]\times7\times62,500=581,875$$ Thus, the net exchange rate will be: $$\frac{581,875}{437,500}=USD\ 1.33$$
The above result can be explained as follows:
The exchange rate rises, and hence the hedge improves (deteriorates) the price by USD 0.02 per GBP (=1.32-1.30). However, note that the basis is USD 0.03 (=1.35-1.32) per GBP. The basis amount implies that the company can cash out the futures contract at USD 1.32 per GBP and buy the underlying asset at USD 1.35 per GBP. Given that there is no basis risk, the company will pay the future price of USD 1.30. However, the basis risk increases the futures price to USD 1.33.
There are instances when it may be impossible to find futures contracts on a particular underlying asset. In such scenarios, the hedger may turn to futures on securities that positively correlate with the underlying. This is called cross hedging. In other words, cross hedging involves hedging the risk exposure of one asset with the futures contracts in another asset. Since the assets are not entirely identical, there must be enough correlation for the hedge to work. The Hedge Ratio is the proportion of position in the futures contracts to the position in the underlying asset.
In cross hedging analysis, the relationship between the changes in spot price and futures price during the life of the hedge is considered. As such, define the following:
\(\Delta S\) = change in the spot price during the life of the hedge.
\(\Delta F\) = change in the futures price during the life of the hedge.
The above variables are regressed using the historical data, and that the line of best fit is given by:
$$\Delta S=a+b\Delta F+\epsilon$$
Where \(a\) and \(b\) are constant, and \(\epsilon\) is an error term.
Now define the variable h as the hedge ratio such that the proportional change in the value per given unit hedged asset is given by:
$$\Delta S-h\Delta F=a+\left(b-h\right)\Delta F+\epsilon$$
If we let h =b, then the above equation reduces to:
$$\Delta S-h\Delta F=a+\epsilon$$
The optimal hedge ratio, also called the minimum variance ratio, is the degree of correlation between the underlying asset and the futures contract purchased to hedge financial risks. It is the ratio of the futures position to the spot position.
Define the following variables:
\(\rho\) = correlation coefficient between \(\Delta S\) and \(\Delta F\).
\(\sigma_S\) = standard deviation of \(\Delta S.\)
\(\sigma_F\) = standard deviation of \(\Delta F\).
\(h^0\) = Optimal hedge ratio.
Using the result from the cross-hedging analysis, recall that the slope coefficient in a linear model is given by:
$$b=\frac{cov(\Delta S,\Delta F)}{\sigma_{\Delta F}^2}=\frac{\rho\sigma_{\Delta S}\sigma_{\Delta F}}{\sigma_{\Delta F}^2}=\rho\frac{\sigma_{\Delta S}}{\sigma_{\Delta F}}$$
Using the above analogy and the defined variables, it is true that:
$$h^0=\rho\frac{\sigma_S}{\sigma_F}$$
The last expression implies that the hedge effectiveness is a proportion of \(\Delta S\) that is hedged away and usually termed as \(R^2\) of the regression. In the case of a linear model with one variable, \(R^2=\rho^2\).
The variables \(\rho\),\(\sigma_S\), and \(\sigma_F\) are usually determined from the historical data over the life of the hedge. However, short periods are normally used to get more reliable estimates.
Define the following variables:
\(Q_A\) = number of units of the position being hedged.
\(Q_F\) = number of units of underlying assets in one futures contract.
\(N^0\) = Optimal number of futures needed for hedging.
The optimal number of futures contracts for hedging a given exposure s given by:
$$N^0=\frac{h^0Q_A}{Q_F}$$
Example: Determining the Optimal Number of Futures Contracts Needed to Hedge an Exposure
A US airline wishes to hedge its jet fuel using heating oil. From the historical data, it is estimated that the correlation between the monthly price changes of heating oil prices and jet fuel prices is 0.65. The standard deviation of monthly changes in heating oil futures prices is $0.045 per gallon, and that of jet fuel price per gallon is $0.034 per gallon.
The airline has also estimated that it will purchase 2 million gallons of jet fuel in one month. As such, the airline wishes to hedge this position. As per the exchange contract terms, one futures contract is on 50,000 gallons of heating oil.
Calculate the optimal hedge ratio and the number of long contracts required for the airline to hedge its position.
Solution
The optimal hedge ratio is given by: $$h^0=\rho\frac{\sigma_S}{\sigma_F}=0.65\times\frac{0.034}{0.045}=0.4911\approx 49.11\%$$
The number of long contracts needed by the airline to hedge its position is given by: $$N^0=\frac{h^0Q_A}{Q_F}=\frac{0.4911\times2,000,000}{50,000}=19.64\approx20$$
The hedging analysis presented thus far is true when forward contracts are considered. However, if we are to use futures contracts, we ought to make an adjustment called tailing the hedge to accommodate the fact that the futures are settled daily (the hedger uses a series of daily hedges). Typically, analysts will use the standard deviation of the daily returns rather than the standard deviation of price changes.
Define the following variables:
\({\hat{\sigma}}_S\) = standard deviation of one-day returns in the spot price, equivalent to the percentage change in the spot price.
\({\hat{\sigma}}_F\) = standard deviation of one-day returns as provided by the futures price and equivalent to a percentage change in futures.
\(\hat{\rho}\) = the correlation coefficient between the one-day spot and futures returns.
\(S\) = Spot price.
\(F\) = Futures price.
\(Q_A\) = number of units of the asset being hedged.
\(Q_F\) = number of units of underlying assets on one futures contract.
\(V_A = SQ_A\) = value of the position being hedged.
\(V_F = FQ_F\) = value of one futures contract.
From the above variables, it is easy to see that:
$$\text{Standard deviation of daily spot price change} = \hat{\sigma}_SS$$ and$$\text{Standard deviation of futures price change} = \(\hat{\sigma}_FF\) $$Thus, the optimal hedge portfolio is given by:$$h^0=\hat{\rho}\frac{{\hat{\sigma}}_SS}{{\hat{\sigma}}_FF}$$Consequently, the optimal number of futures contracts needed to hedge an exposure is given by
$$N^0=\hat{\rho}\frac{{\hat{\sigma}}_SS}{{\hat{\sigma}}_FF}\frac{Q_A}{Q_F}=\hat{\rho}\frac{{\hat{\sigma}}_SV_A}{{\hat{\sigma}}_FV_F}$$
The optimal hedge ratio and the optimal number of contracts needed to hedge an exposure is estimated as:
$${\hat{h}}^0=\hat{\rho}\frac{{\hat{\sigma}}_S}{{\hat{\sigma}}_F}$$
And
$$N^0={\hat{h}}^0\frac{V_A}{V_F}$$
Note: Identify the differences between these formulas and the ones in cross hedging analysis given as:
$$h^o=\rho\frac{\sigma_S}{\sigma_F}$$
Notice that \(\sigma_S\) and \(\sigma_F\) are the standard deviation of changes in \(S\) and \(F\), respectively over the life of the hedge, and \(\rho\) is the correlation between \(\sigma_S\) and \(\sigma_F\). On the other hand, \({\hat{\sigma}}_S\) and \({\hat{\sigma}}_F\) are the standard deviation of daily returns in \(S\) and \(F\), respectively and \(\hat{\rho}\) is the correlation coefficient between \({\hat{\sigma}}_S\) and \({\hat{\sigma}}_F\).
Example: Tailing the Hedge
A risk manager notices that the standard deviation of daily return on spot price is 1.5% in a given exchange, and the standard deviation of daily return on futures price is 0.9%. The correlation between the daily returns on the spot and futures price is 0.75. The risk manager wishes to hedge assets worth $100,000. The value of one futures contract is $10,000.
What is the optimal hedge ratio and the optimal number of contracts needed by the risk manager to hedge its exposure?
Solution
The optimal hedge ratio is given by: $${\hat{h}}^0=\hat{\rho}\frac{{\hat{\sigma}}_S}{{\hat{\sigma}}_F}=0.75\times\frac{0.015}{0.009}=1.25$$
The optimal contracts are given by: $$N^0={\hat{h}}^0\frac{V_A}{V_F}=1.25
\times\frac{100,000}{10,000}=13$$
Stock index futures can be used to alter exposure in the market. In other words, stock index futures provide a way of increasing or decreasing the market exposure without paying a lot in transaction fees. For instance, consider an investor who is excellent at choosing stocks but has no opinion on the market’s future course. The investor will wish to construct a portfolio and then hedge the market’s return to earn an excess return of the selected stocks over the market’s return.
Example: Hedging Equity Position
An investor has a diversified portfolio of $360,000. The portfolio is set such that it closely tracts FTSE 100. The investor wants to have three-month market exposure. To hedge the investor’s position, the investor uses the CME futures contracts on FTSE 100, which is $60 multiplied by the index. The futures price is $3,000.
Assume that the standard deviation of the daily return on the futures price and the standard deviation of the daily return of the asset being hedged is equal. Moreover, the correlation between the daily returns of the spot and futures price is 1.0.
What is the optimal number of contracts to hedge the investor’s position?
Solution
Given the information given in the question, we have that: $$N^0=\frac{V_A}{V_F}=\frac{360,000}{60\times3,000}=2$$
As defined in the capital asset pricing model, beta is a measure of a portfolio’s systematic risk. When a trader uses index futures to hedge a position in an equity portfolio, they effectively reduce its systematic risk. As such, hedging is an attempt to reduce a portfolio’s beta.
Assume that we want to use S&P 500 futures to hedge a well-diversified portfolio with a beta \(\beta\). By assuming that the standard deviation of the daily return of the asset being hedged is equal to \(\beta\) multiplied by the standard deviation of the return provided by the futures price and the correlation between the daily return on the asset and the futures price is approximately 1.0, then the futures contracts to hedge a position is given by:
$$N^0=\beta\frac{V_A}{V_F}$$
Example: Calculating Optimal Contracts using Stock Index Futures
An investor contracts a portfolio with a beta of 1.45 and worth $300,000. The six-month index futures are worth $3,000 per contract. The index futures contract is on $60 multiplied by the index.
What is the optimal number of contracts required to hedge the portfolio?
Solution
The optimal number of contracts is given by: $$N^0=\beta\frac{V_A}{V_F}=1.45\times\frac{300,000}{60\times3,000}=2.42\approx2$$
The example above assumes that the investor wishes to hedge the beta entirely, that is, to reduce the beta to zero. However, index futures can also be used to modify the portfolio beta. Let us define the following variables:
\(\beta\) = current portfolio beta.
\(\beta^\ast\) = target beta.
\(V_A\) = value of the position being hedged.
\(V_F\) = value of one futures contract.
If \(\beta > \beta^\ast \) the number of contracts that the investor should short is given by:
$$\left(\beta-\beta^\ast\right)\frac{V_A}{V_F}$$
On the other hand, if \(\beta<\beta^\ast\) the number of contracts needed by the investor to take a long position in is given by:
$$\left(\beta^\ast-\beta\right)\frac{V_A}{V_F}$$
Example: Modifying Portfolio Beta
An investor contracts a portfolio with a beta of 1.45 and worth $600,000. The six-month index futures are worth $3,000 per contract. The index futures contract is on $60 multiplied by the index.
The investor wishes to reduce the portfolio beta to 0.65. What is the required number of contracts that the investor needs to short?
Solution
Based on the information given in the question, \(\beta = 1.45\) and \(\beta^\ast=0.65\); thus, the required number of contracts is given by: $$\left(\beta-\beta^\ast\right)\frac{V_A}{V_F}=\left(1.45-0.65\right)\frac{600,000}{3,000\times60}=2.667\approx3$$
The investor, therefore, needs to short three futures contracts to reduce the portfolio beta from 1.45 to 0.65.
Constructing Long-Term Hedges using Stack and Roll Strategy
Hedgers are sometimes confronted with a scarcity of liquid futures contracts for the desired hedge maturities since most liquid futures contracts usually have shorter maturities. To overcome this challenge, the hedgers employ a stack and roll strategy.
The stack and roll strategy involves the following:
Example: Stack and Roll Strategy
An oil refinery company knows that it will need to sell 1 million barrels of refined oil in 18 months. It is currently 1 January of the first year. Based on the exchange to which the company is registered, the liquid futures contracts are those with maturities of 6 months or less. However, the company wishes to construct a long-term hedge using a stack and roll strategy.
The exchange’s refined oil futures contract trade with maturities in February, April, June, July, October, and December. The futures contract is traded on 5000 barrels of oil per contract. To implement its strategy, the company does the following:
The futures price at each subsequent closeout and purchase of futures contracts is as follows:
What is the total profit(loss) on the short positions of the futures contracts?
Solution
Based on the information given in the question, the profit per contract if given by:
$$5,000\left(56-55\right)+5,000\left(55.50-53\right)+5,000\left(54-57\right)=$2,500$$
Thus, the total gain is:
$$\text{Total Gain}=\frac{1,000,000}{5,000}\times2,500=$500,000$$
Because of the nature of the daily settlements of futures, discrepancies may arise between the cash flows in a futures contract used for hedging and the ones from the exposure that is being hedged. Therefore, care should be taken to ensure that losses from a futures contract can be easily financed until gains from the hedged positions start to flow in.
Question 1
Rachel Zane, FRM, is a portfolio manager in charge of a $200 million, well-diversified portfolio that has a beta of 1.5 relative to the Nasdaq-100. The current value of the 3-month Nasdaq-100 Index is 2,500, and the multiplier is 300. Over the next three months, Ms. Zane wants to use the Nasdaq-100 futures to reduce the systematic risk of the portfolio to 1.0. To pull that off, which of the following moves is required?
- Sell 100 contracts
- Buy 133 contracts
- Sell 133 contracts
- Buy 100 contracts
The correct answer is C.
Number of contracts required=\(\left( { \beta }^{ \ast }-\beta \right) \left( \frac { P }{ A } \right) \)
Where:
\(\beta\)=portfolio beta
\( { \beta }^{ \ast }\)=target beta after hedging
\(P\)=portfolio value
\(A\)=value of futures contract
$$ \begin{align*}&=1.0-1.5\times \frac { 200,000,000 }{ 2,500\times 300 }\\ &=-133\\ \end{align*}$$
The negative sign implies 133 contracts need to be sold.
Question 2
Which of the following accurately explains the differences between a normal and an inverted futures market in terms of hedging strategies, storage costs, market expectations, and convenience yields?
A. In a normal market, futures prices are higher than spot prices due to higher storage costs, while in an inverted market, futures prices are lower than spot prices because of higher convenience yields.
B. In a normal market, futures prices are lower than spot prices due to lower storage costs, while in an inverted market, futures prices are higher than spot prices because of higher market expectations.
C. In a normal market, futures prices are higher than spot prices due to higher convenience yields, while in an inverted market, futures prices are lower than spot prices because of lower market expectations.
D. In a normal market, futures prices are lower than spot prices due to higher convenience yields, while in an inverted market, futures prices are higher than spot prices because of lower storage costs.
Solution
The correct answer is A.
In a normal futures market, futures prices are generally higher than spot prices because the carrying costs, including storage, are incorporated into the price. Higher storage costs would lead to higher futures prices relative to spot prices. The convenience yield, which represents the benefits of physically holding a commodity, is typically lower.
In an inverted futures market, the situation reverses. The futures prices are lower than spot prices, indicating an expectation of a tighter spot market in the future. This scenario often results from anticipated shortages or high demand, leading to higher convenience yields.
B is incorrect because in a normal market, futures prices are higher, not lower than spot prices, due to carrying costs, including storage costs. In an inverted market, futures prices are lower, not higher than spot prices. The relationship between futures and spot prices in both normal and inverted markets is incorrectly represented.
C is incorrect because the description of a normal market is incorrect. Higher convenience yields would typically be associated with an inverted market, not a normal market. Additionally, this choice doesn’t accurately capture the relationships between storage costs and convenience yields.
D is incorrect because this choice fails to accurately represent the dynamics between futures and spot prices in both normal and inverted markets. In a normal market, futures prices are generally higher, not lower than spot prices, and the relationship between convenience yields and storage costs is misrepresented.
Things to Remember
- In a normal futures market, carrying costs, such as storage, are a significant factor and result in futures prices being higher than spot prices.
- In an inverted futures market, futures prices are less than spot prices. Higher convenience yields often cause this situation, signaling a tighter spot market in the future.
- Convenience yield refers to the benefits of physically holding a commodity and plays a key role in understanding the pricing of futures markets.
- The relationships between spot prices, futures prices, storage costs, and convenience yields are fundamental in comprehending market expectations and the structure of both normal and inverted futures markets.