Measures of Central Tendency and Location
The center of any data is identified via a measure of central tendency. A measure of central tendency for a series of returns reveals the center of the empirical distribution of returns. They include mean, mode, and median. Measures of…
Other Return Measures
Other Return Measures Gross and Net Return The gross return is what an asset manager earns before subtracting various costs such as management fees, custody fees, taxes, and other administrative expenses. However, it does account for trading costs such as…
Annualized Returns
To compare returns over different timeframes, we need to annualize them. This means converting daily, weekly, monthly, or quarterly returns into annual figures. Non-Annual Compounding Interest may be paid semiannually, quarterly, monthly, or even daily – interest payments can be…
Money-weighted and Time-weighted Rates of Return
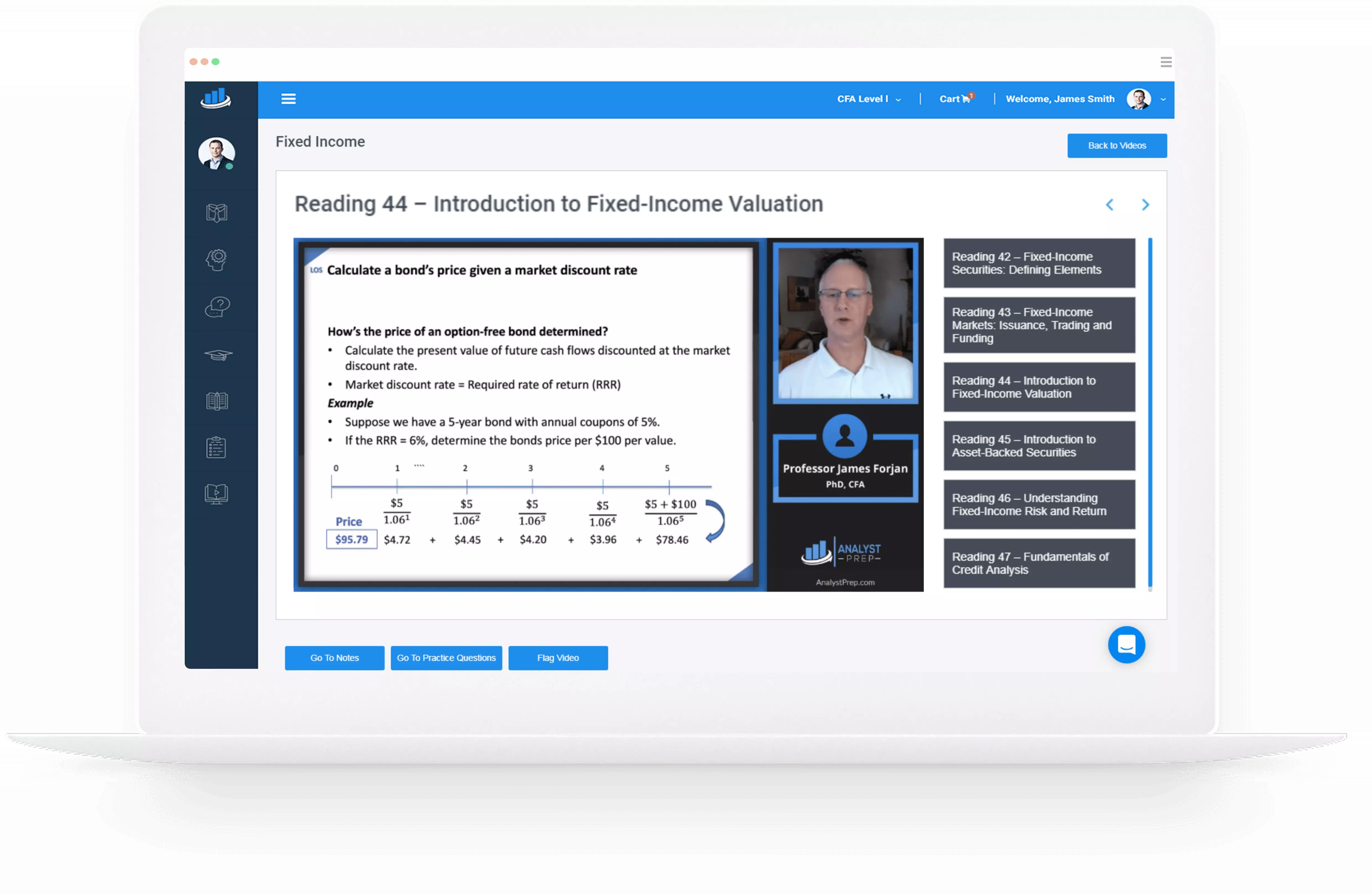
Money-weighted Rate of Return The money-weighted return considers the money invested and gives the investor information on the actual investment return. Calculating money-weighted return is similar to calculating an investment’s internal rate of return (IRR). The money-weighted rate of return…
Measures of Return
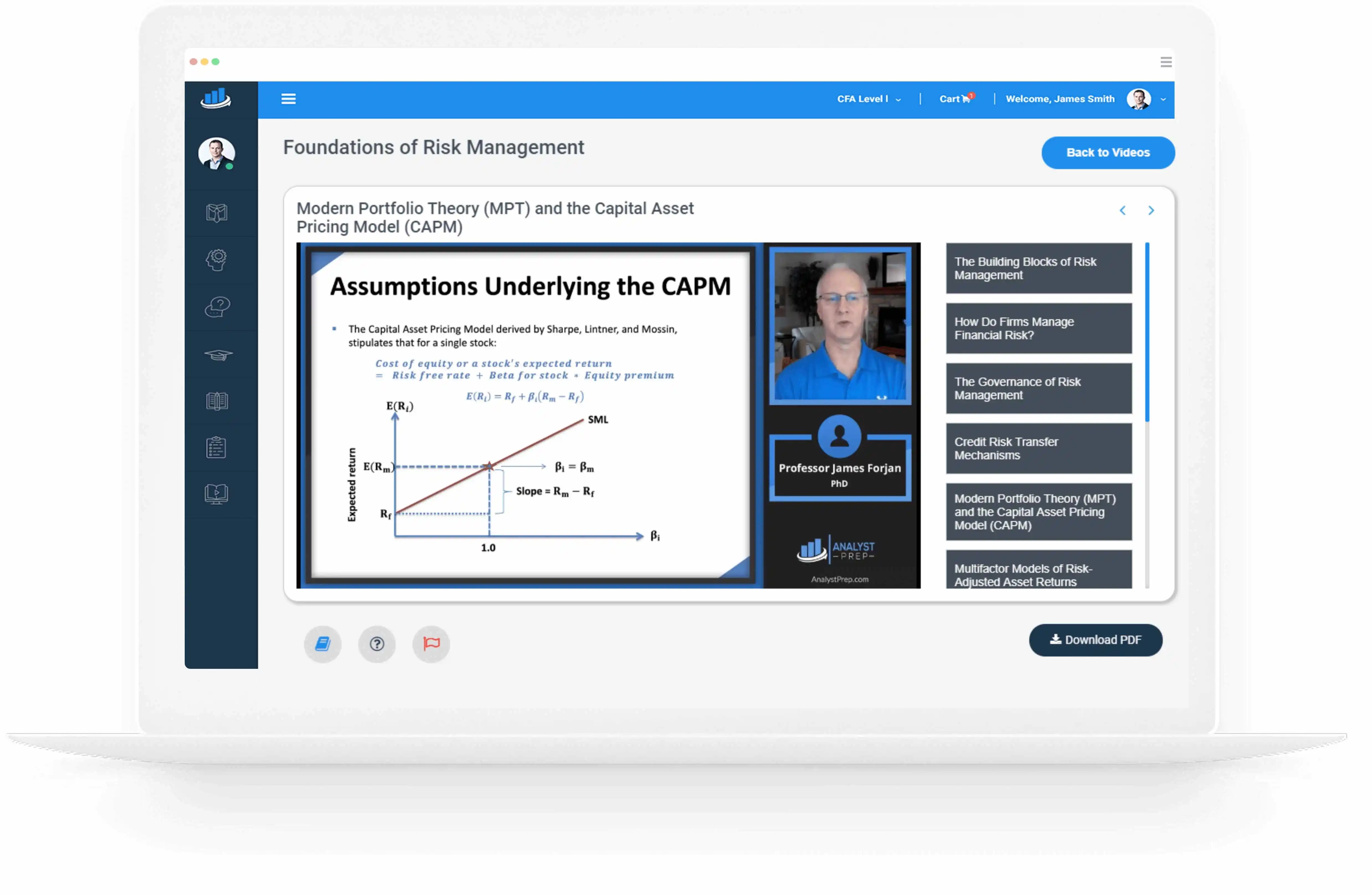
Financial assets are primarily defined based on their return-risk characteristics. This definition approach helps when building a portfolio from all the assets available. It’s noteworthy that there are different ways of measuring returns. Financial market assets generate two types of…
Interest Rates
The time value of money is a concept that states that cash received today is more valuable than cash received in the future. If a person agrees to receive payment in the future, he foregoes the option of earning interest…
Risk-Neutrality in Derivative Pricing
Remember that the value of an option is not affected by the real-world probabilities of the underlying price increments or decrements but rather by the expected volatilities (\(R^u\) and \(R^d\) ), which are required to price an option. We can…
One-Period Binomial Model
The law of arbitrage dictates that the value of any two assets (or portfolio of assets) whose payoffs are identical in all possible future scenarios at a given time must also be identical today. Unlike forward commitments that offer symmetric…
Put-Call Forward Parity
The put-call forward parity extends the put-call parity to include the forward contracts. To get the put-call forward parity, we substitute the present value of the forward price, \(F_0(T)\), for the underlying price:$$F_0(T)\left(1+r\right)^{-T}+p_0=c_0+X\left(1+r\right)^{-T}$$ Deriving Put-Call Forward Parity Consider an investor…
Put-Call Parity
Put-call parity is a no-arbitrage concept. It involves a combination of cash and derivative instruments in a portfolio. Put-call parity allows pricing and valuation of these positions without directly modeling them using non-arbitrage conditions. Deriving Put-Call Parity Consider an investor…