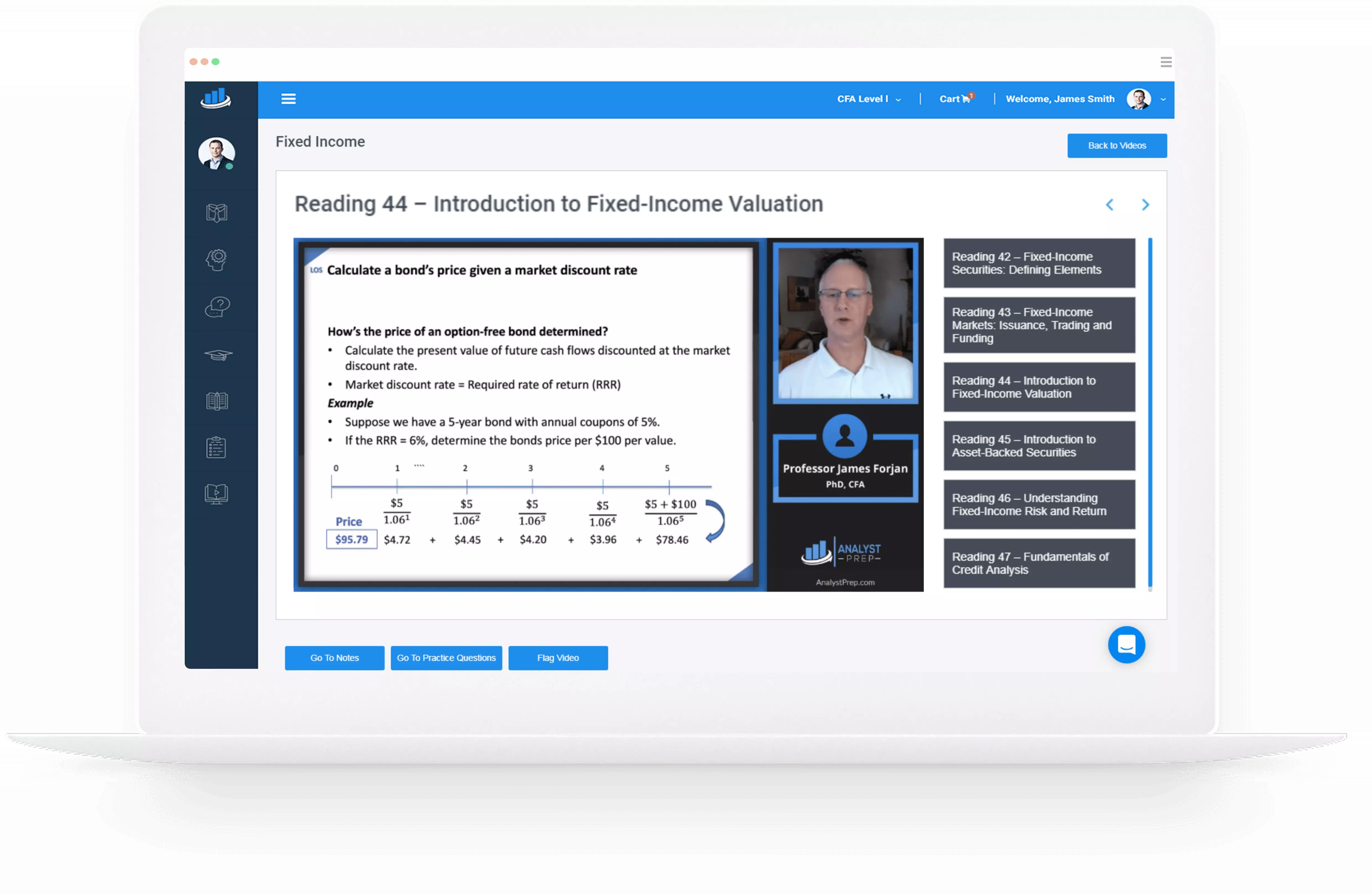
Binomial Option Valuation Model
One-Period Binomial Option Valuation Model In the one-period binomial model, we start today (at time t=0) when the stock price is \(S_{0}\). Then, the stock price can either jump upwards or downwards over the one-period time interval to t=1. This…
Study Notes for CFA® Level II – Derivatives – offered by AnalystPrep
Reading 37: Pricing and Valuation of Forward Commitments -a. Describe and compare how equity, interest rate, fixed-income, and currency forward and futures contracts are priced and valued; –b. Calculate and interpret the no-arbitrage value of equity, interest rate, fixed-income, and…
Pricing and Valuation of Interest Rate Swaps
Swaps are typically derivative contracts in which two parties exchange (swap) cash flows or other financial instruments over multiple periods for a give-and-take benefit, usually to manage risk. Both swap contract parties have future obligations. Thus, similar to forwards and…
Pricing and Valuation Concepts
A forward commitment is a derivative contract that allows one to buy or sell an underlying security at a predetermined price at a future date. The price of a forward or a futures contract is the prespecified price that the…
Choosing the Appropriate Time-Series Model
The following guidelines are used to determine the most appropriate model depending on the need: Understand the investment problem. This is followed by choosing the initial model. Plot the time series to check for covariance stationarity. Observe if there is…
Cointegration
Consider a time series of the inflation rate \((\text{y}_{\text{t}})\) regressed on a time series of interest rates \((\text{x}_{\text{t}})\): $$\text{y}_{\text{t}}=\text{b}_{0}+\text{b}_{1}\text{x}_{\text{t}}+\epsilon_{\text{t}}$$ In this case, we have two different time series, \(\text{y}_{\text{t}}\) and \(\text{x}_{\text{t}}\). Either one of the time series is subject to…
Autoregressive Conditional Heteroskedasticity
Heteroskedasticity is the dependence of the variance of the error term on the independent variable. We have been assuming that time series follows the homoskedasticity assumption. Homoskedasticity is the independence of the variance of the error term on the independent…
Seasonality
Seasonality is a time series feature in which data shows regular and predictable patterns that recur every year. For example, retail sales tend to peak for the Christmas season and then decline after the holidays. A seasonal lag is the…
The Unit Root Test for Nonstationary
Unit root testing involves checking whether the time series is covariance stationary. We can either form an AR model and check for autocorrelations or perform a Dickey and Fuller test. A t-test is performed to examine the statistical significance of…
Unit Roots for Time-Series Analysis
The Unit Root Problem An AR(1) series is said to be covariance stationary if the absolute value of the lag coefficient \(\text{b}_{1}\) is less than 1. If the absolute value of \(\text{b}_{1}=1\), the time series is said to have a…