Calculate conditional probabilities
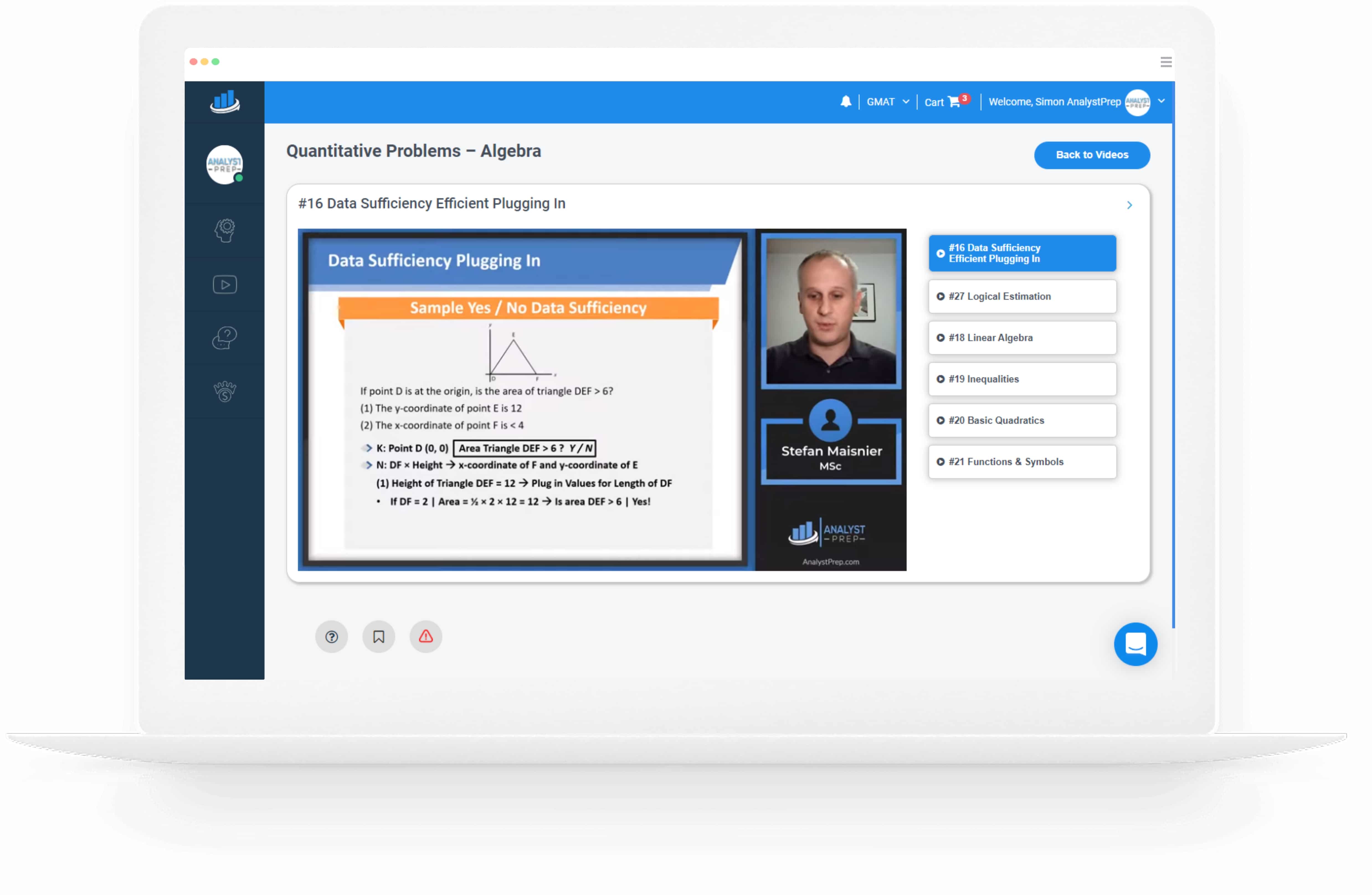
While it's crucial to grasp basic probability concepts, actuarial problems often require a deeper dive into complex scenarios where events are interdependent. This leads us to the concept of conditional probability. Conditional probability is the probability of an event occurring,…
Calculate the expected value, variance, and standard deviation of both the loss random variable and the corresponding payment random variable upon the application of policy adjustments.
In the previous reading, we covered the fundamental ideas of deductibles, coinsurance, benefit limits, and inflation in the context of insurance. In this reading, we will focus on more quantitative aspects. Specifically, we will perform calculations to determine the expected…
Apply the concepts of deductibles, coinsurance, benefit limits, and inflation to convert a given loss amount from a policyholder into the corresponding payment amount for an insurance company
Policy modifications refer to changes made to the loss random variable for an insurance product. In this chapter, we will explore several policy modifications, each serving a specific purpose in enhancing insurance coverage. These modifications include: Deductibles Benefit/policy limits Coinsurance…
Apply Transformations
Transformations allow us to find the distribution of a function of random variables. There are different methods of applying transformations. The Method of Distribution Function Given a random variable \(Y\) that is a function of a random variable \(X\), that…
Determine the sum of independent random variables (Poisson and normal)
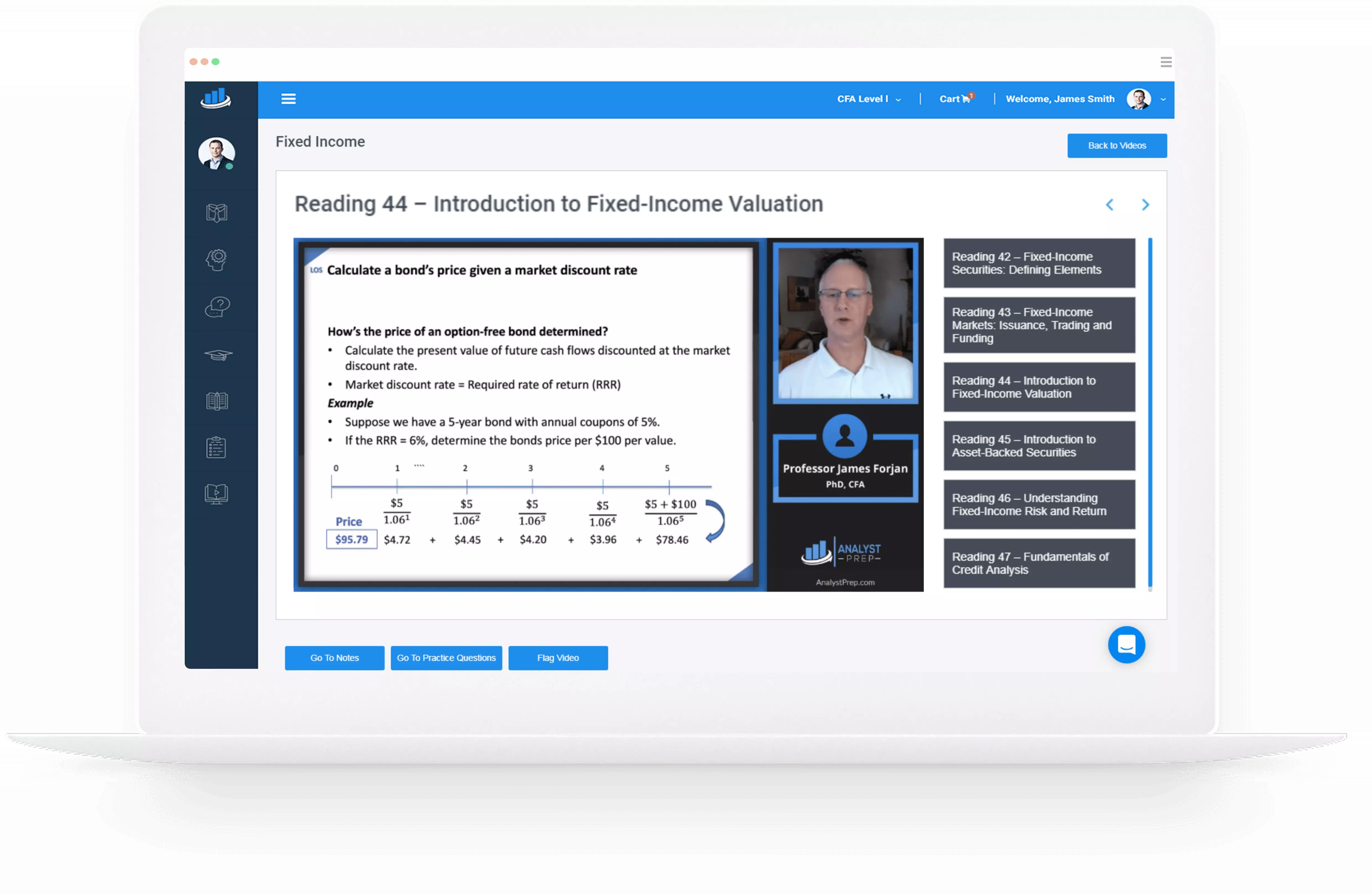
The Sum of Independent Random Variables Given \(X\) and \(Y\) are independent random variables, then the probability density function of \(a=X+Y\) can be shown by the equation below: $$ { f }_{ X+Y }\left( a \right) =\int _{ -\infty }^{…
Probability Generating Functions and Moment Generating Functions
Probability Generating Function The probability generating function of a discrete random variable is a power series representation of the random variable’s probability density function as shown in the formula below: $$ \begin{align*} \text{G}\left(\text{n}\right)&=\text{P}\ \left(\text{X}\ =\ 0\right)\bullet \ \text{n}^0\ +\ \text{P}\…
Explain and calculate variance, standard deviation, and coefficient of variation
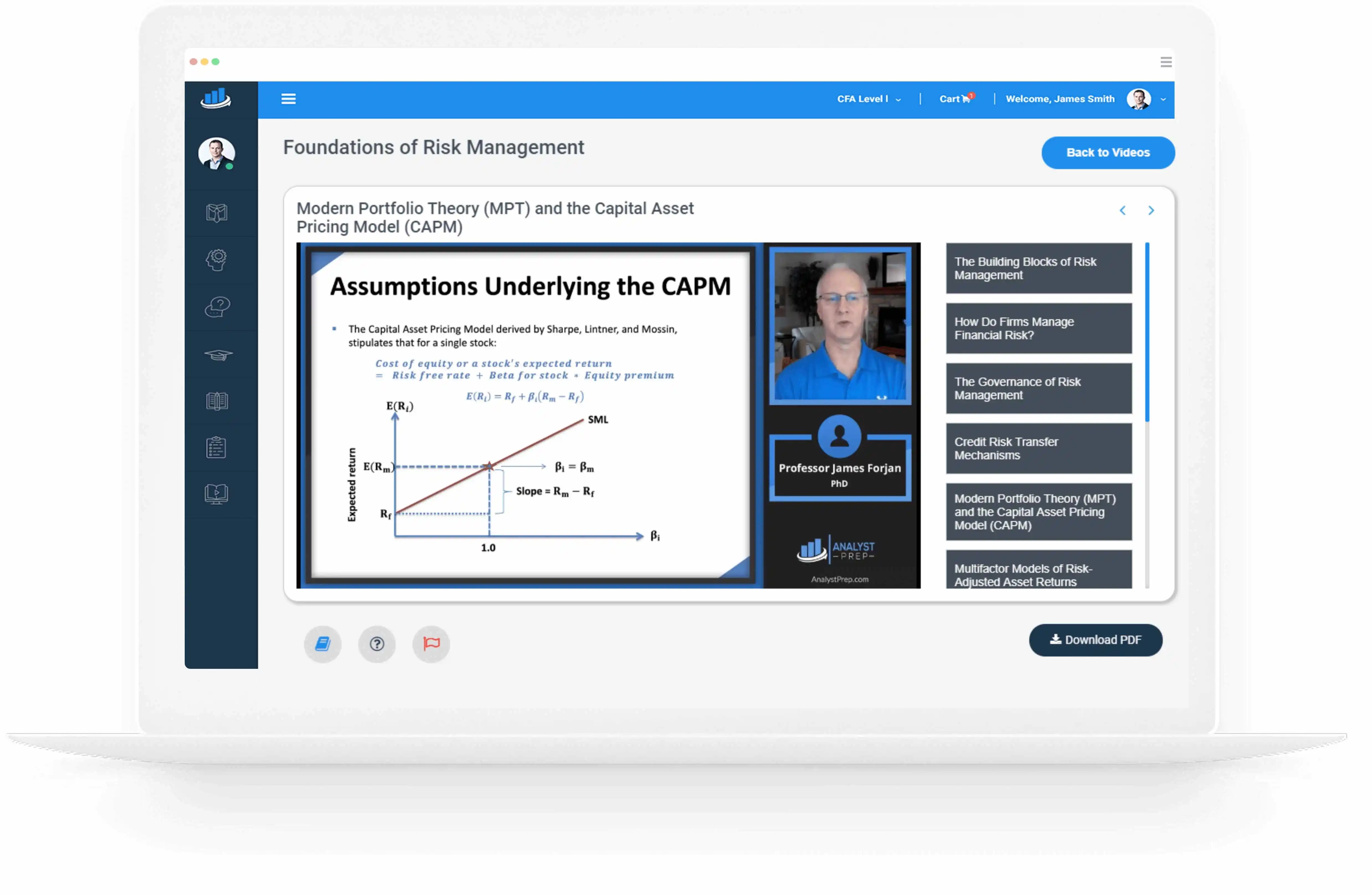
Variance The mean (average) gives an idea of the “typical” value in a dataset. However, in many scenarios, especially in financial markets, simply knowing the mean isn’t sufficient. Investors want to know not just the expected return (mean) but also…
Explain and calculate expected value and higher moments, mode, median, and percentile
Expected Value of Discrete Random Variables Let \(X\) be a discrete random variable with probability mass function, \(p(x)\). The expected value or the mean of the random variable \(X\), denoted as \(E(X)\), is given by: $$ E \left(X\right)=\sum{x. p (x)}…
Explain and apply the concepts of random variables
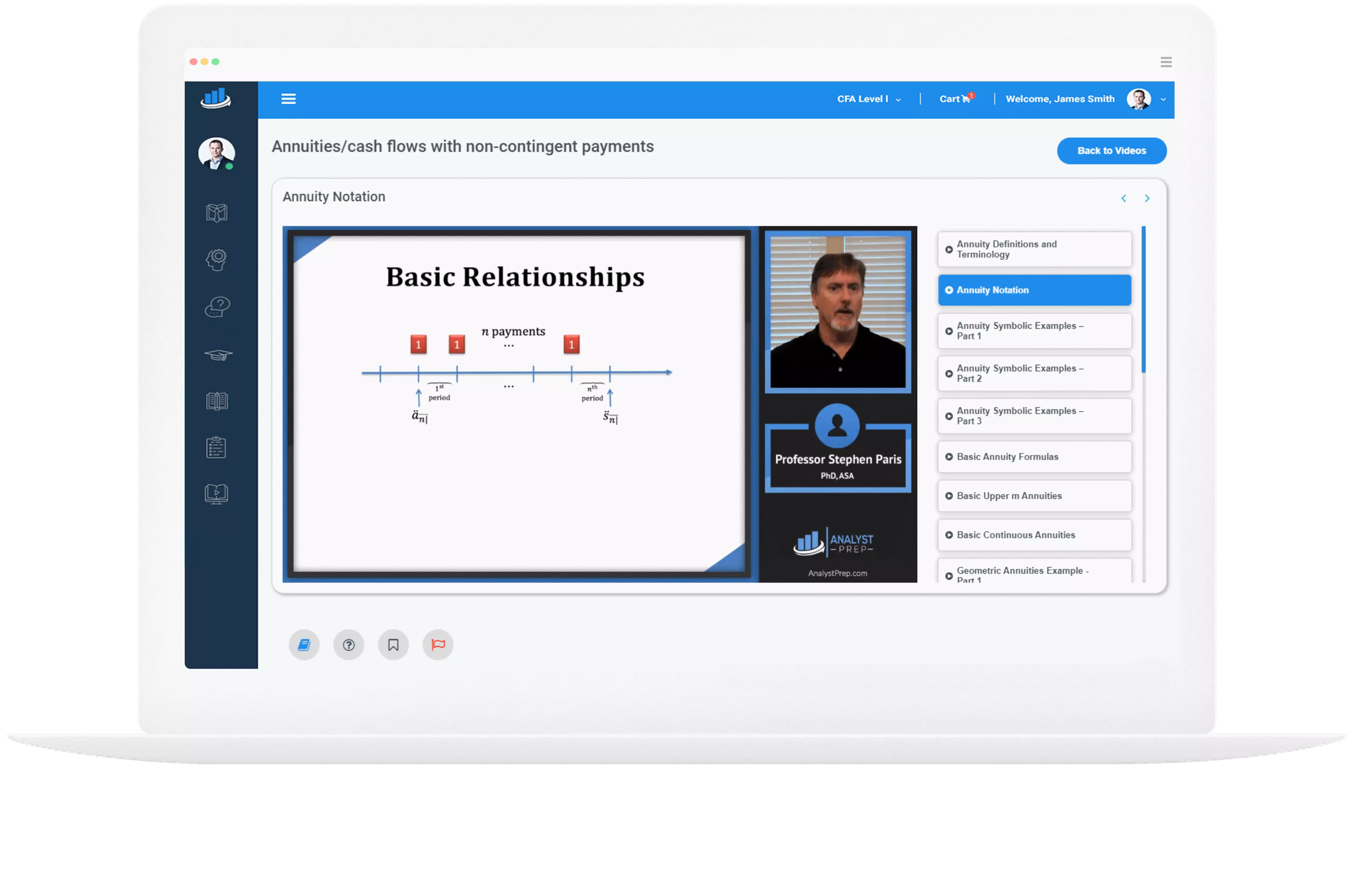
Definitions: Variable: In statistics, a variable is a characteristic, number, or quantity that can be measured or counted. Random variable: A random variable (RV) is a variable that can take on different values, each with a certain probability. It essentially…