Pricing using the Zero-Coupon Yield Curve and an Arbitrage-Free Binomial Lattice

Valuing a fixed-rate coupon bond with no embedded options using the arbitrage-free lattice and the spot curve leads to the same bond value. This holds because the binomial interest rate tree is arbitrage-free. However, the spot curve will not work for bonds with embedded options.

Example: Zero-Coupon Yield Curve

A three-year bond with no embedded options pays 5% annual coupons. Given the following spot curve, the bond price with a face value of $100 is closest to:

$$ \begin{array}{c|c} \textbf{Term to Maturity} & \textbf{Spot Rate} \\ \hline 1 & 4.00\% \\ \hline 2 & 5.00\% \\ \hline 3 & 6.00\% \end{array} $$

Solution

$$ \begin{align*} PV &=\frac{PMT}{\left(1+S_1\right)^1}+\frac{PMT}{\left(1+S_2\right)^2}+\ldots+\frac{PMT+FV}{\left(1+S_N\right)^N} \\  &=\frac{5}{1.04}+\frac{5}{{1.05}^2}+\frac{105}{{1.06}^3}=$97.50 \end{align*} $$

Example: Arbitrage-Free Binomial Lattice

We can determine the price of the same bond using a binomial interest rate tree with the following forward rates:

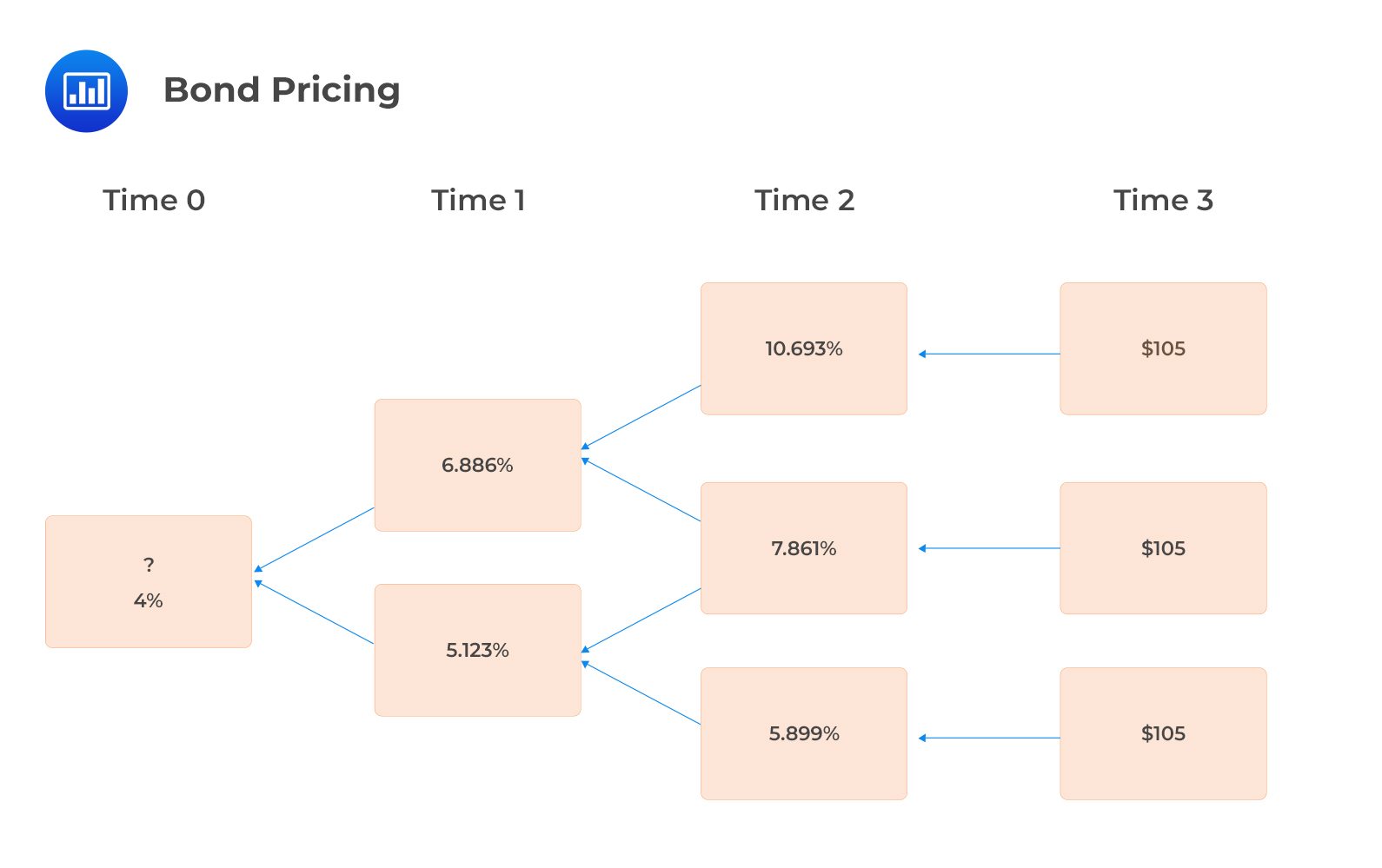Solution

$$ \begin{align*} V &=0.5\left[\frac{V_u+C}{1+i}+\frac{V_d+C}{1+i}\right] \\ V_{2,uu} & =\frac{105}{1.10693}=94.857 \\ V_{2,ud} &=\frac{105}{1.07861}=97.348 \\ V_{2,dd} &=\frac{105}{1.05899}=99.151 \\ V_{1,u} &=0.5\left[\frac{94.857+5}{1.06886}+\frac{97.348+5}{1.06886}\right]=94.589 \\ V_{1,d} &=0.5\left[\frac{97.348+5}{1.05123}+\frac{99.151+5}{1.05123}\right]=98.218 \\ V_0&=0.5\left[\frac{94.589+5}{1.04}+\frac{98.218+5}{1.04}\right]=$97.50 \end{align*} $$

We have calibrated the binomial interest rate tree to produce arbitrage-free values consistent with the spot rate curve. This has led to the same option-free bond value of $97.50 as using the spot yield curve.

Question

A fixed-rate coupon bond with no embedded options which is priced using the arbitrage-free binomial lattice leads to a value that’s:

1. Higher than that generated using the benchmark spot rate curve.
2. Lower than that generated using the benchmark spot rate curve.
3. Similar to that generated using the benchmark spot rate curve.

Solution

The correct answer is C.

Valuing a fixed-rate coupon bond with no embedded options using the arbitrage-free lattice and using the spot curve leads to the same bond value. This holds because the binomial interest rate tree is arbitrage-free.

Reading 29: The Arbitrage-Free Valuation Framework

LOS 29 (f) Compare pricing using the zero-coupon yield curve with pricing using an arbitrage-free binomial lattice.