Chi-square and F-Distributions
Chi-square Distribution A chi-square distribution is an asymmetrical family of distributions. A chi-square distribution with \(v\) degrees of freedom is the distribution of the sum of the squares of \(v\) independent standard normally distributed random variables. Intuitively, chi-square distributions take…
Monte Carlo Simulation
Monte Carlo simulations involve the creation of a computer-based model into which variabilities and interrelationships between random variables are entered. A spread of results is obtained when the model is run hundreds or thousands of times. This explains why this…
t-Distribution and Degrees of Freedom
A student’s t-distribution is a bell-shaped probability distribution symmetrical about its mean. It is regarded as the most suitable distribution to use in the construction of confidence intervals in the following instances:
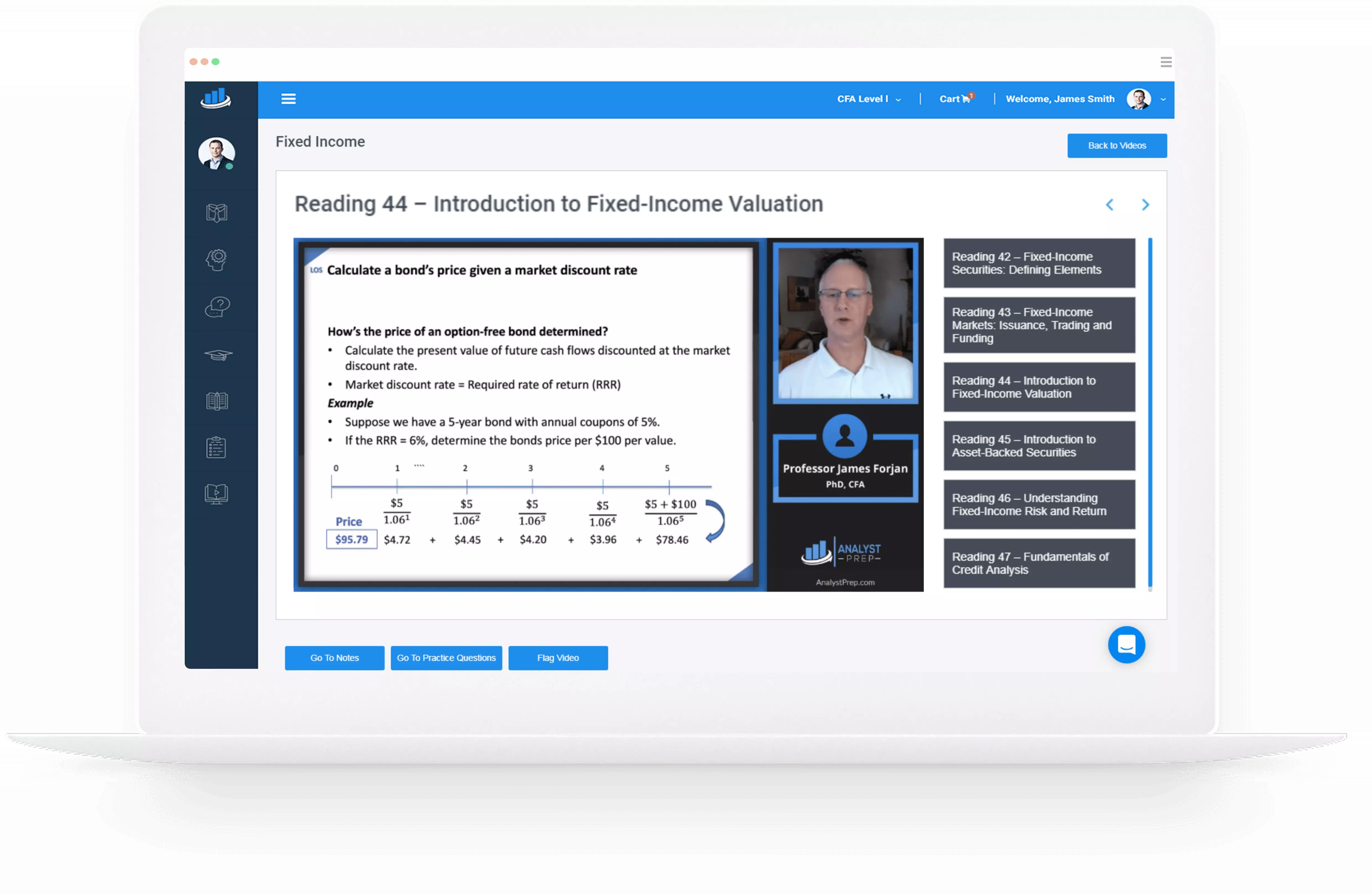
Continuously Compounded Rate of Return Given Holding Period Return
Continuous compounding applies either when the frequency with at we calculate interest is infinitely large or the time interval is infinitely small. Put quite simply, under continuous compounding, time is viewed as continuous. This is a departure from discrete compounding,…
Relationship between Normal Distribution and Lognormal Distribution
A variable \(X\) is said to have a lognormal distribution if \(Y = ln(X)\) is normally distributed, where “ln” denotes the natural logarithm. In other words, when the logarithms of values form a normal distribution, we say that the original…
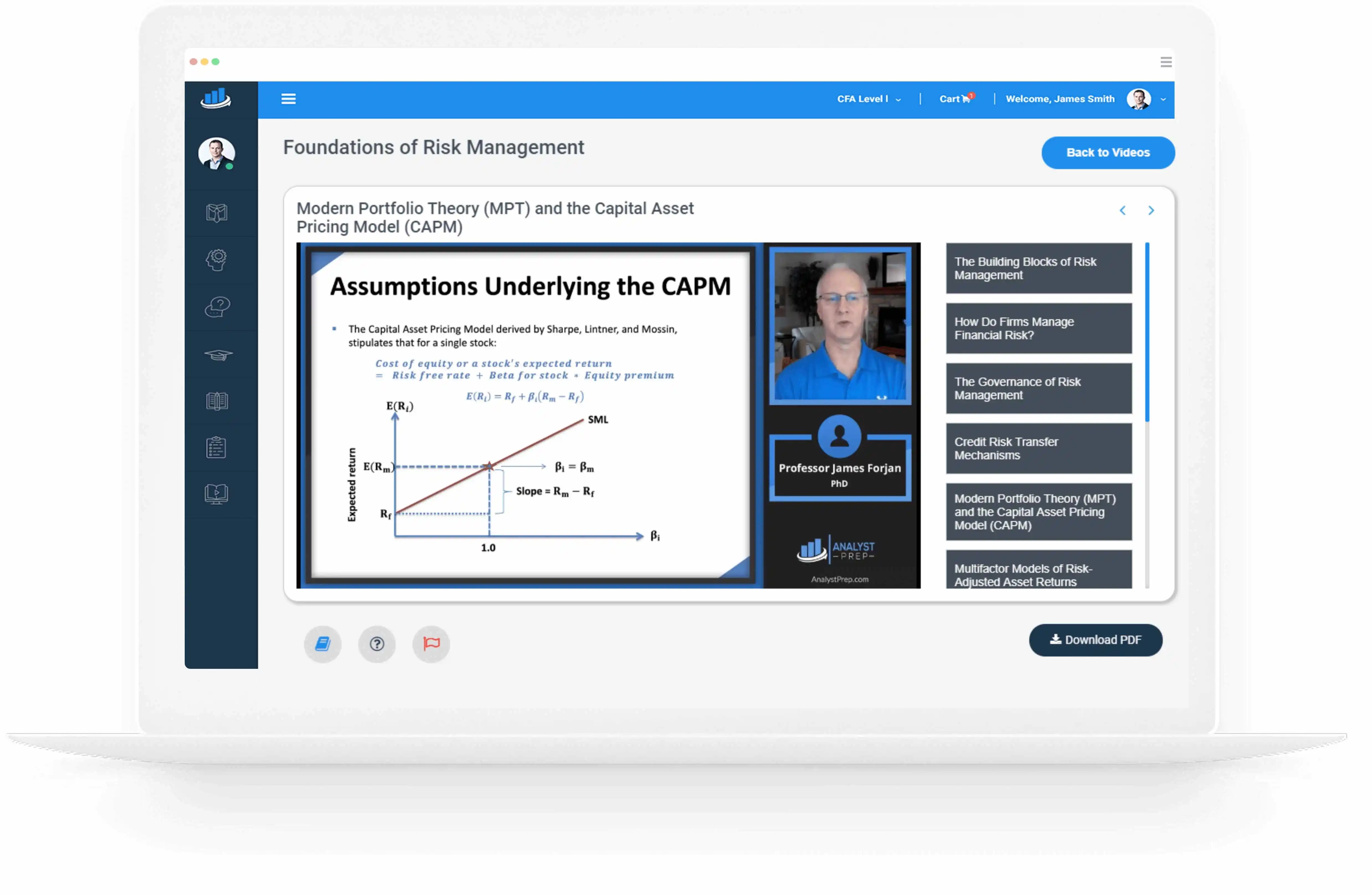
Shortfall Risk, Safety-first Ratio, and Identification of an Optimal Portfolio Using Roy’s Safety-first Criterion
Shortfall risk refers to the probability that a portfolio will not exceed the minimum (benchmark) return that an investor has set. In other words, it is the risk that a portfolio will fall short of the level of return an…
Using the Standard Normal Distribution to Calculate Probabilities
Using the standard normal distribution table, we can confirm that a normally distributed random variable \(Z\), with a mean equal to 0 and variance equal to 1, is less than or equal to \(z\), i.e., \(P(Z ≤ z)\). However, the…
The Standard Normal Distribution
The standard normal distribution refers to a normal distribution that has been standardized such that it has a mean of 0 and a standard deviation of 1. The shorthand notation used is: $$ N \sim (0, 1) $$ In the…
Normal Distribution and Confidence Intervals
A confidence interval (CI) gives an “interval estimate” of an unknown population parameter, such as the mean. It gives us the probability that the parameter lies within the stated interval (range). The precision or accuracy of the estimate depends on…
Univariate Distribution, Multivariate Distribution, and Correlation
Univariate and multivariate normal distributions are very robust and useful in most statistical procedures. Understanding their form and function will help you learn a lot about most statistical routines.