Use of Leveraged Loans.

In fixed-income instruments, leveraged loans, high-yield bonds, and convertible bonds hold a unique position. These instruments, characterized by either fixed or variable-rate coupons, offer specific benefits and risks to both private market issuers and investors throughout the investment life cycle. They also incorporate contingency features and spreads against comparable risk-free government securities. These spreads serve to compensate investors for the probability of issuer default, loss severity if a default occurs, and relative liquidity.

Credit spreads, the difference in yield between a U.S. Treasury bond and another debt security of the same maturity but different credit quality, can vary significantly across issuers, seniority rank, and time periods. For instance, the liquidity of Apple Inc.’s bonds tends to be higher than that of a smaller, less-known company’s leveraged loans. In contrast to investment-grade loans and bonds, such as those issued by Microsoft Corporation, half or more of the total yield-to-maturity of high-yield bonds is often attributable to yield spread for private market issuers, which fall within the sub-investment grade category.

High-yield bonds and convertible bonds are used in private market strategies. However, most private debt professionals and investors do not consider them as part of the private debt asset class. For example, a private equity firm might use high-yield bonds to finance a leveraged buyout, but this would not typically be considered a private debt strategy.

Leveraged Loans

Leveraged loans are a type of loan that is often used by companies with a high level of debt or poor credit history. They are typically used for large-scale transactions such as mergers and acquisitions, buyouts, or real estate purchases. These loans have unique features and calculations that differentiate them from other types of loans.

Features of Leveraged Loans

• Leveraged loans are a type of floating-rate loan, where the debt coupons fluctuate over time as the Market Reference Rate (MRR) changes. For instance, if a company borrows a leveraged loan with a floating rate tied to the LIBOR, the interest rate on the loan will change as the LIBOR rate changes.
• The issuer-specific credit spread is usually fixed for the entire loan term. This means that the risk premium charged to the borrower remains constant throughout the loan term.
• As base rates adjust to market conditions, the price of a leveraged loan remains close to par if market credit spreads and issuer credit quality are unchanged. This means that the loan’s price will not significantly fluctuate with changes in market interest rates.
• Leveraged loans provide issuers greater flexibility than other term financing alternatives due to their interest rate feature and the right to prepay loans at par. This means that the borrower can repay the loan before its maturity without any prepayment penalty.
• These loans are commonly used in private buyout situations and for acquisition financing where the loan is secured by target company assets. For example, in a leveraged buyout (LBO), the acquiring company can use the assets of the target company as collateral for the loan.
• The MRR on a leveraged loan often sets at the beginning of an interest period with payment at the end, which may be monthly, quarterly, or semiannually. This means that the interest rate on the loan is reset at the beginning of each interest period based on the prevailing MRR.

Calculating Leveraged Loan Price

The leveraged loan price (PV) is considered without accrued interest on a given rate reset date. This means that the price of the loan is calculated based on the principal amount and not the accrued interest. The quoted margin (QM) established at the time of loan issuance compensates investors for issuer credit risk. This is the additional interest charged to the borrower to compensate for the risk of default. The required margin (or discount margin (DM)) is the market-determined yield spread over or under the MRR such that the leveraged loan is priced at par on a reset date. This is the additional yield required by the market to hold the loan.

• Leveraged loan price for fixed rate: The price of a fixed-rate, non-callable bond on a coupon date with the bond’s future or par value of future value (FV), market discount rate r, and coupon payment PMT for N years is calculated using the formula:

  $$ \begin{align*} PV = \frac{PMT}{(1+r)} + \frac{PMT}{(1+r)^2} + \cdots + \frac{PMT + FV}{(1+r)^N} \end{align*} $$

  Fixed-rate loans are ideal when you need consistent payments over the life of the loan. This predictability aids in budgeting and financial planning, particularly for individuals or businesses with fixed income or cash flow. If you anticipate that interest rates will rise in the future, locking in a fixed rate can save money over the long term. It protects you from paying higher interest costs as rates increase.

• Leveraged loan price for floating rate: For a floating-rate loan, the numerator of the present value calculation changes each period as a function of the MRR and the quoted margin, while the market discount rate in the denominator depends on the MRR and the market-driven DM.

  $$ \begin{align*} PV & = \frac{(MRR + QM) \times FV}{(1 + \frac{MRR_m + DM}{m})} + \frac{(MRR + QM) \times FV}{\left(1 + \frac{MRR_m + DM}{m}\right)^2} \\ & + \cdots + \frac{(MRR + QM) \times FV + FV}{\left(1 + \frac{MRR_m + DM}{m}\right)^N} \end{align*} $$

  Floating rates often start lower than fixed rates, making them attractive in the short term. This can be beneficial for borrowers who plan to pay off their loan quickly or expect to refinance in the near future. If you expect interest rates to decrease, a floating-rate loan allows you to benefit from lower payments as rates drop. This could be due to economic predictions or upcoming monetary policy changes.

Interest Rate Exposure

• An investor faces far less exposure to interest rate risk under a floating rate structure but remains exposed to credit spread risk. This means that while the investor is protected from changes in interest rates, they are still exposed to the risk of the borrower’s creditworthiness deteriorating.
• Changing short-term MRR rates result in adjusted debt coupons payable by leveraged loan issuers to investors, while bond investors receive a constant coupon while facing capital gains (losses) in a lower (higher) benchmark rate environment. This means that the interest payments on leveraged loans adjust with changes in market rates, while bond investors face the risk of price changes due to interest rate fluctuations.
• The quoted versus discount margin on a leveraged loan is an important determinant of its price on a reset date. This means that the difference between the quoted margin and the discount margin can significantly impact the price of the loan.

Structural Flexibility of Leveraged Loans

• Leveraged loans allow borrowers under a private buyout strategy to tailor debt terms to match business plan milestones. For example, a company undertaking a LBO can negotiate the terms of the loan to align with the projected cash flows of the target company.
• Examples include the renegotiation of covenants, extension of loan tenor, or setting a variable credit spread based upon financial leverage or coverage criteria targets to increase debt capacity or in exchange for fewer restrictive covenants. For instance, a company may negotiate for a lower credit spread if it achieves certain financial targets.
• Fixed-rate bond terms are more standardized and difficult to amend, with bond indenture changes often requiring the consent of a majority of bondholders to make changes. This means that the terms of a bond are more rigid and cannot be easily changed to suit the borrower’s needs.

In leveraged loans, pricing can vary significantly based on the market’s perception of risk and value. The terms ‘Par’, ‘Discount’, and ‘Premium’ are crucial for investors to understand, as they directly impact investment returns. ‘Par’ refers to a loan priced at its nominal value, ‘Discount’ indicates a loan priced below its nominal value, offering a potential value buy, and ‘Premium’ signifies a loan priced above its nominal value, often reflecting lower perceived risk or high demand.

$$ \begin{array}{l|l|l}
{\text{Leveraged} \\ \text{Loan Price}} & \text{Description} & {\text{Quoted} \\ \text{Versus} \\ \text{Discount} \\ \text{Margin}} \\ \hline
\text{Par Pricing} & { \text{Loan trades at a price (present value) equal to} \\ \text{its future value. This scenario typically} \\ \text{reflects a balanced view of risk and reward,} \\ \text{indicating that the loan is priced in line with} \\ \text{its perceived risk.}} & {QM = DM} \\ \hline
\text{Discount} & {\text{Loan trades at a price below its future value.} \\ \text{This might indicate that the loan is perceived} \\ \text{to carry higher risk or there are expectations of} \\ \text{interest rates rising. Purchasing at a discount} \\ \text{can offer higher returns if the perceived risk} \\ \text{does not materialize.}} & {QM \lt DM} \\ \hline
\text{Premium} & {\text{Loan trades at a price above its future value.} \\ \text{This typically occurs when the loan is seen} \\ \text{as lower risk or in high demand. While buying} \\ \text{at a premium might result in lower yields, it} \\ \text{often reflects a safer investment choice.} } & {QM \gt DM}
\end{array} $$

High-yield bonds

• High-yield bonds, also known as junk bonds, are fixed-rate debt instruments issued by sub-investment grade borrowers.
• Typically issued for terms of 5 to 10 years, often on a subordinated basis.
• Common issuers include startup companies without long credit histories.
• Issued at holding company level, providing greater liquidity due to higher disclosure requirements and standardization.

An indenture is a legal document outlining the terms, conditions, and covenants of the bond. High-yield bonds usually lack maintenance covenants but have restrictive and incurrence covenants. A key restrictive covenant is the change of control provision, protecting investors if a significant ownership change occurs.

Change of Control Clause

• Requires the issuer to offer to repurchase bonds at or above par if there’s a significant change in ownership.
• Gives bondholders the right to sell back bonds or renegotiate terms under new ownership that could affect their returns.

Callable Bonds

• High-yield bonds may include a call feature allowing issuers to repurchase bonds after a specified period, typically at a premium.
• This feature impacts the bond’s pricing, as it includes the cost of the issuer’s option to call the bond.

$$\text{Callable bond value} = \text{Straight bond value} – \text{issuer call option value}$$

Bond Valuation

• The value of a non-callable bond is calculated by discounting its future cash flows at the yield-to-maturity or appropriate discount rates.

Compare callable high-yield to non-callable bonds

Practitioners typically evaluate risky bonds using either an issuer-specific yield curve with borrowing rates over different tenors, or a series of default risk-free zero rates plus a constant spread estimated using the market prices of comparable bonds for issuers of similar credit quality, known as the zero-volatility spread (Z-spread) , while also taking credit spread volatility into account.

The option-adjusted spread (OAS) is a generalization of the Z-spread calculation that incorporates bond option pricing based on assumed interest rate volatility. The OAS measure is the constant yield spread over the zero curve, which makes the arbitrage-free value of a bond equal to its market price, allowing comparisons across callable, putable, and non-callable bonds.

Price–Yield Relationship for Leveraged Loans and High-Yield Bonds

The characteristics of the price–yield relationship are crucial for understanding the dynamics of leveraged loans and high-yield bonds in the financial markets, distinguishing them from default risk-free government bonds and investment-grade bonds.

• Fixed-rate bonds’ price sensitivity to changes in benchmark yield as shown in the figure below includes:
  • A negative, linear first-order duration component.
  • A positive, non-linear second-order convexity component.
• The price–yield relationship differs significantly for leveraged loans and high-yield bonds when compared to risk-free or investment-grade bonds due to the uncertainty of future cash flows.

Effective Duration (EffDur)

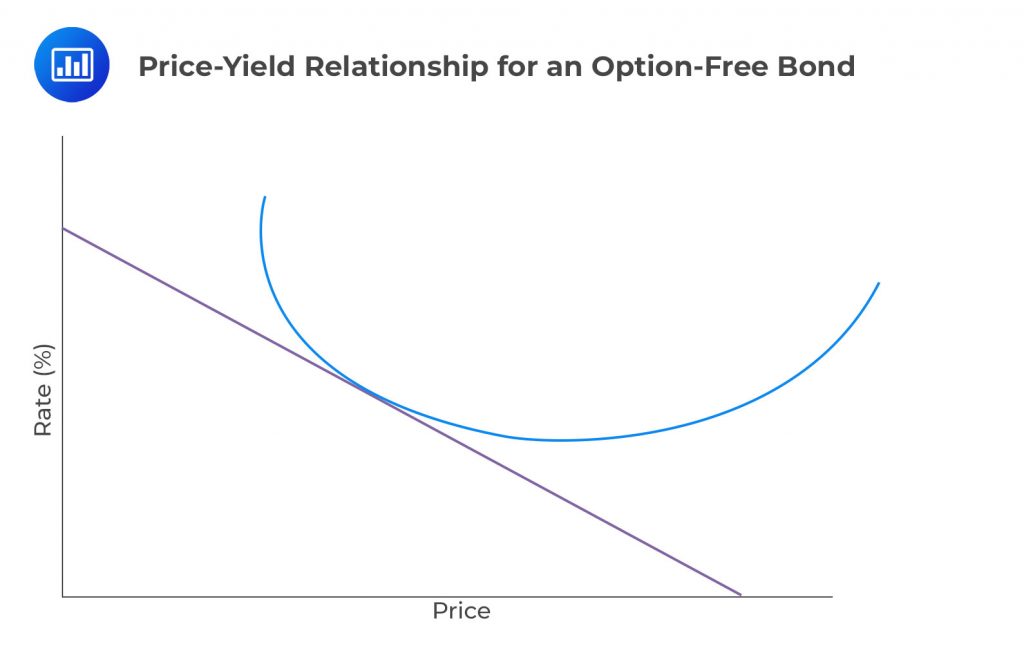
For bonds with uncertain cash flows, effective duration measures interest rate sensitivity:

$$\text{Effective Duration (EffDur)} = \frac{(PV_{+}) – (PV_{-})}{2 \times (\Delta \text{Curve}) \times (PV_0)}$$

Where:

• \(PV_{+}\) represents the bond’s price if the interest rate decreases (i.e., the price of the bond if the yield curve shifts downwards).
• \(PV_{-}\) represents the bond’s price if the interest rate increases (i.e., the price of the bond if the yield curve shifts upwards).
• \(\Delta \text{Curve}\) is the change in the yield curve, representing an instantaneous parallel shift in the issuer-specific yield curve.
• \(PV_0\) is the initial price of the bond or loan before the shift in the yield curve.

Above shows how the price of a bond or loan responds to changes in the interest rate environment, factored by an average of the price increase and decrease divided by the change in yield, adjusted for the initial price of the bond. It provides a numeric value representing the years it takes for a bond to repay its cost, adjusted for changes in interest rates.

By measuring the bond’s price sensitivity to interest rate changes through Effective Duration, investors can better gauge the potential volatility and interest rate risk of bonds with uncertain cash flows.

High-Yield Bonds vs. Non-Callable Bonds

• A non-callable bond typically has a higher price than its callable high-yield counterpart due to the value of the call option.
• Low interest rates relative to the bond’s coupon increase the likelihood of the issuer refinancing at lower rates.

Price–Yield Sensitivity of Leveraged Loans

Leveraged loans, being floating-rate, have a different sensitivity to yield changes:

$$\text{EffSpreadDur} = \frac{(PV_{+}) – (PV_{-})}{2 \times (\Delta \text{Spread}) \times (PV_0)}$$

Where:

• \(\Delta \text{Spread}\) represents the change in the yield spread, which is the difference in yield between the leveraged loan and a benchmark rate.

It determines how the price of a leveraged loan responds to changes in its yield spread relative to the market or a benchmark. \(\text{EffSpreadDur}\) is especially relevant for floating-rate instruments like leveraged loans, where interest rate changes affect the yield spread rather than the base rate itself. It measures the sensitivity of a leveraged loan’s price to changes in the yield spread, indicating the expected percentage change in price for a 1% change in the spread.

• The effective duration of non-callable fixed-rate bonds exceeds that of high-yield bonds due to the issuer’s call option value increasing as yields decrease.
• Leveraged loans’ price sensitivity is directly tied to yield spread changes, not overall yield changes, due to periodic MRR resets based on market conditions.
• Market conditions, such as issuer financial distress or a “flight to quality,” can significantly impact the price movement of high-yield bonds beyond what benchmark rate and credit spread models might predict.

Convertible Bonds

Convertible bonds are a unique type of financial instrument that combines the features of both debt and equity. They are essentially long-term fixed-income instruments that provide investors with the option to convert the debt into equity at a predetermined conversion price per share during a specified future conversion period. The value of a convertible bond is intrinsically linked to the issuer’s share price and is calculated as the sum of the value of a straight bond and the value of a call option on the issuer’s stock.

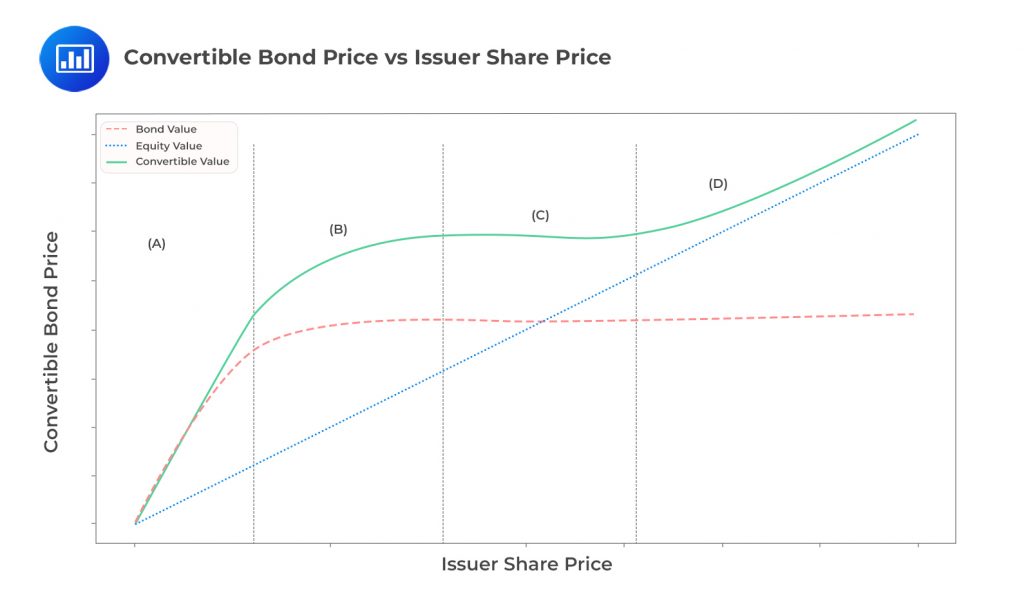
These bonds exhibit fixed income characteristics when the conversion feature is unlikely to be exercised, such as when the issuer’s share price is significantly below the conversion price. However, they take on equity-like features as the issuer’s shares rise to near or above the price at which conversion occurs. To better understand the value of convertible bonds, we can categorize them into four quadrants based on the issuer’s share price and the likelihood of conversion:

• Quadrant A: This is a distressed case where the values of the convertible and straight bond are nearly equal and the equity value is close to zero. An example could be a startup company facing financial difficulties.
• Quadrant B: In this quadrant, the convertible bond’s value is more closely aligned with expected interest and principal payments. This could be a mature company with stable cash flows.
• Quadrant C: This is a hybrid case where the share price is near the conversion price. An example could be a growth company with increasing share price.
• Quadrant D: As the issuer share price rises above the conversion price, the convertible bond price approaches the equity value. This could be a high-growth company with rapidly increasing share price.

Convertible bonds are more common in early-stage and growth strategies for private companies. Issuers may raise debt through convertible bonds as a low-cost alternative to issuing common shares. These bonds offer equity upside in the form of conversion rights to investors who might otherwise be unwilling to lend to firms with less stable cash flows and few assets to secure debt. Convertible debt is typically senior unsecured with no financial covenants and a debt coupon well below that of non-callable debt.

Convertible bonds issued by private companies are less liquid and typically in the form of privately placed convertible notes. Common convertible bond indenture terms include change of control protection and the right to trigger conversion at a discounted price to capture the effects of dilution in the case of new equity financing.

Conversion Ratio and Conversion Value

The conversion ratio is a key term in convertible bonds. It represents the number of common shares a bond may be converted into for a specific par value. It is calculated as the convertible bond par value divided by the conversion price. For example, if a bond with a par value of $1000 can be converted into 50 shares, the conversion ratio is 50.

The conversion value is another important concept. It is derived by comparing the convertible bond’s price with its value if the bondholder were to exchange bonds for shares today. It is calculated as the conversion ratio multiplied by the current share price. For instance, if the conversion ratio is 50 and the current share price is $20, the conversion value is $1000.

Practice Questions

Question 1: Leveraged loans, high-yield bonds, and convertible bonds are all fixed-income instruments that offer unique benefits and risks to both issuers and investors. Which of the following statements is most accurate about the yield-to-maturity of high-yield bonds for private market issuers?

1. Half or more of the total yield-to-maturity of high-yield bonds is often attributable to the principal amount.
2. Half or more of the total yield-to-maturity of high-yield bonds is often attributable to yield spread.
3. Half or more of the total yield-to-maturity of high-yield bonds is often attributable to the coupon rate.

Answer: Choice B is correct.

Half or more of the total yield-to-maturity of high-yield bonds is often attributable to yield spread. High-yield bonds, also known as junk bonds, are bonds that are rated below investment grade by credit rating agencies. They carry a higher risk of default than investment-grade bonds, and therefore, they offer higher yields to compensate investors for the additional risk. The yield-to-maturity (YTM) of a bond is the total return that an investor would receive if the bond is held until maturity. It includes both the coupon payments and any capital gain or loss that would result from selling the bond at its current market price. The yield spread is the difference between the YTM of a high-yield bond and the YTM of a comparable risk-free government bond. It represents the additional yield that an investor requires for taking on the higher risk of a high-yield bond. Therefore, it is accurate to say that half or more of the total YTM of high-yield bonds is often attributable to the yield spread.

Choice A is incorrect. The principal amount of a bond does not contribute to its yield-to-maturity. The principal amount is the face value of the bond that is returned to the investor at maturity. It does not change over the life of the bond and does not provide any return to the investor.

Choice C is incorrect. While the coupon rate is a component of the yield-to-maturity of a bond, it is not accurate to say that half or more of the total YTM of high-yield bonds is often attributable to the coupon rate. The coupon rate is the annual interest payment that the bond issuer pays to the bondholder, expressed as a percentage of the bond’s face value. However, the YTM of a bond also includes any capital gain or loss that would result from selling the bond at its current market price, as well as the yield spread over a comparable risk-free government bond. Therefore, the coupon rate alone does not account for half or more of the total YTM of high-yield bonds.

Question 2: Leveraged loans, high-yield bonds, and convertible bonds are all types of debt instruments. Each of these instruments has unique features and can be used in different investment strategies. In private market strategies, which of the following statements is most accurate?

1. Most private debt professionals and investors consider high-yield bonds and convertible bonds as part of the private debt asset class.
2. Most private debt professionals and investors do not consider high-yield bonds and convertible bonds as part of the private debt asset class.
3. Most private debt professionals and investors consider only leveraged loans as part of the private debt asset class.

Answer: Choice B is correct.

Most private debt professionals and investors do not consider high-yield bonds and convertible bonds as part of the private debt asset class. Private debt refers to loans or other debt instruments that are not publicly traded on an exchange. These are typically issued by private companies or by public companies as private placements. High-yield bonds and convertible bonds, on the other hand, are typically issued by public companies and are traded on public exchanges. Therefore, they are not considered part of the private debt asset class. Private debt is characterized by its illiquidity, higher potential returns, and higher risk compared to public debt. It is typically used by institutional investors and high-net-worth individuals as part of a diversified investment strategy.

Choice A is incorrect. As explained above, high-yield bonds and convertible bonds are typically not considered part of the private debt asset class because they are publicly traded. While they may offer higher yields than investment-grade bonds, they do not have the same characteristics as private debt.

Choice C is incorrect. While leveraged loans are often part of the private debt asset class, they are not the only type of instrument in this category. Other types of private debt can include direct lending, mezzanine debt, distressed debt, and other types of non-bank lending. Therefore, it is not accurate to say that most private debt professionals and investors consider only leveraged loans as part of the private debt asset class.

Glossary

• Leveraged Loans: A type of loan that is extended to companies or individuals that already have considerable amounts of debt.
• High-Yield Bonds: A high paying bond with a lower credit rating than investment-grade corporate bonds, Treasury bonds, and municipal bonds.
• Convertible Bonds: A type of bond that the holder can convert into a specified number of shares of common stock in the issuing company or cash of equal value.
• Credit Spreads: The spread between Treasury securities and non-Treasury securities that are identical in all respects except for quality rating.
• Unitranche Debt: A type of debt that combines senior and subordinated debt into one debt instrument.
• Convertible Bonds: Long-term fixed-income instruments that allow investors to exchange debt into equity at a predetermined conversion price per share during a future conversion period.
• Conversion Ratio: The number of common shares a bond may be converted into for a specific par value.
• Conversion Value: The value derived by comparing the convertible bond’s price with its value if the bondholder were to exchange bonds for shares today.
• Indenture: A legal and binding agreement, contract, or document between two or more parties.
• Leveraged Loan: A type of loan that is often used by companies with a high level of debt or poor credit history.
• Market Reference Rate (MRR): The benchmark interest rate that the interest rate on a floating-rate loan is tied to.
• Quoted Margin (QM): The additional interest charged to the borrower to compensate for the risk of default.
• Discount Margin (DM): The additional yield required by the market to hold the loan.
• Present Value (PV): The current worth of a future sum of money or stream of cash flows given a specified rate of return.
• Future Value (FV): The value of an asset or cash at a specified date in the future that is equivalent in value to a specified sum today.
• Leveraged Buyout (LBO): The acquisition of another company using a significant amount of borrowed money to meet the cost of acquisition.
• High-yield bonds: Fixed-rate debt instruments issued by sub-investment grade borrowers.
• Indenture: A legal document specifying the terms, conditions, and constraints of a bond.
• Change of control clause: A provision requiring the issuer to offer to repurchase outstanding bonds if a new owner acquires a predetermined percentage of voting shares.
• Callable bond: A bond that the issuer can redeem before its maturity date.
• Z-spread: The constant yield spread over the zero curve that makes the price of a bond equal to its market price.
• Option-adjusted spread (OAS): A measure that incorporates bond option pricing based on assumed interest rate volatility.
• Effective duration: A measure of a bond price’s interest rate sensitivity.

Private Markets Pathway Volume 1: Learning Module 4: Private Debt; LOS 4(b): Discuss the use of leveraged loans, high-yield bonds, and convertible bonds in private market strategies