Replicating Asset Returns Using Options

Position Equivalencies

Options contracts can be utilized to replicate investment positions. When two positions have identical risk exposure and payoff, they are considered equivalent according to the law of one price. This means that they must have the exact cost. By assembling options in a portfolio, synthetic relationships can be formed. These relationships are based on put-call parity, which underlies the connection between the actual positions and their synthetic counterparts.

Put-Call Parity

Put-call parity is a fundamental concept commonly covered in the Level III curriculum. This equation establishes an equilibrium relationship between put and call options prices with the underlying asset. It is crucial to prevent arbitrage opportunities when the market is in balance. To help them remember the components of the equation, candidates often use the mnemonic “sip a coke,” where ‘s’ represents the stock price, ‘p’ represents the price of a put option, ‘a’ represents the equals sign, ‘c’ represents the price of a call option, and ‘k’ represents the strike price shared by both the call and put options (with identical strike prices and expiration dates). The present value of the strike price, denoted as Ke-rt, is sometimes represented as PV(Strike) for visual clarity.

$$ \text{Stock} + \text{Put Option Price} = \text{Call Option Price} + \text{PV(Strike)} $$

This formula may be rearranged to solve for any of the variables and thus serves as a guide in building and creating synthetic positions. It’s important to note that the variables in this equation represent the prices of the put and call options, not their intrinsic value or strike price.

Put-Call Forward Parity

Put-call forward parity is a concept similar to put-call parity, with a slight distinction: instead of the stock position, a long forward contract is used. Candidates can apply the mnemonic “sip a coke” as a helpful tool. They can substitute the underlying stock position with the present value (PV) of holding a long-term contract on the underlying asset. This substitution is utilized in the context of put-call-forward parity. The equation is as follows:

$$ \text{Forward Price} + \text{Put Option Price} = \text{Call Option Price} + \text{PV(Strike)} $$

Synthetic Forwards

Owning a call option and selling a put option with the same strike price and expiration date creates a synthetic long-forward position. This combination is logical because the call option has potential upside gains. On the other hand, the short put option entails the obligation to purchase the asset at the strike price, introducing a downside risk. This setup resembles a forward contract, where the asset must be delivered at the strike price upon expiration. 

$$ \begin{array}{c|c|c} & \textbf{Underlying Increases} & \textbf{Underlying Decreases} \\ \hline \text{Long Forward} & \text{Forward price increases} & \text{Forward price decreases} \\ \hline & \textbf{-OR-} & \\ \hline \text{Long Call} & \text{Call price increases} & \text{Call price decreases} \\ & & \text{toward zero} \\ \hline \text{Short Put} & \text{Put price decreases} & \text{Put price increases} \\ & \text{toward zero} & \\ \end{array} $$

Understanding the nature of a short put as an obligation and a long call as an asset allows us to understand the connection between the synthetic and forward positions. In this case, a synthetic short forward position combines a long put and a short call.

A synthetic long-forward contract is represented below:

Synthetic Put

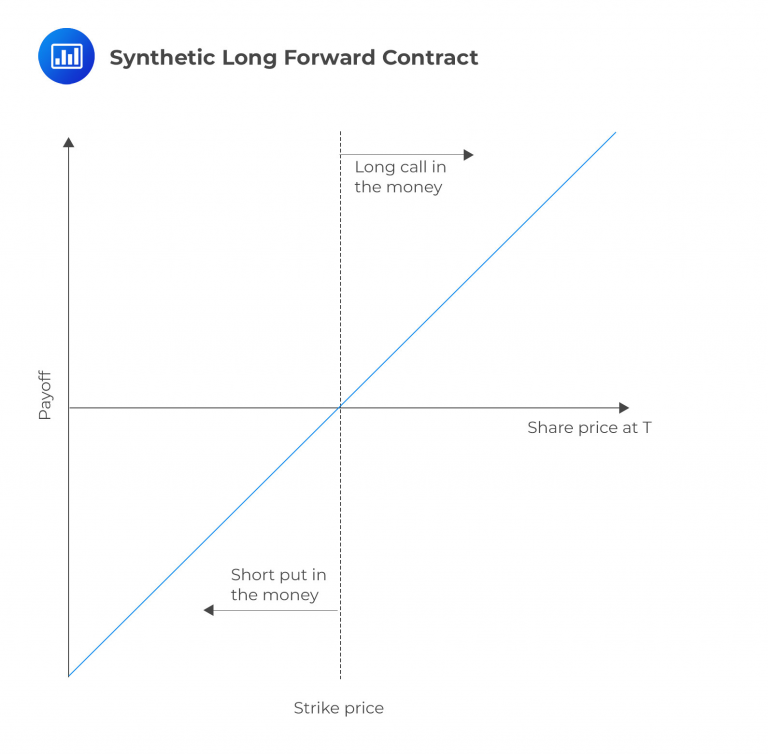
Put-call parity and put-call forward parity serve as a reminder that option positions can be replicated using combinations of the underlying asset and other options. If an investor wishes to replicate a put’s payoff and risk exposures, they would require specific elements. First, since the price of a put increases when the underlying asset declines, the investor needs exposure to downward price movements. Besides, since the price of a put cannot go below zero, a safeguard is necessary. By rearranging put-call parity, the value of a synthetic put can be determined.

$$ \text{Put Option Price} = \text{Call Option Price} + \text{PV(Strike)} – \text{Stock} $$

Synthetic Call

An investor can purchase the underlying asset and a put option to create a synthetic call. Additionally, they need to consider the present value of the strike price, which will determine the payoff equal to the strike at the option’s maturity. The synthetic call can be constructed as follows:

$$ \text{Call Option Value} = \text{Stock} + \text{Put Option Price} – \text{PV(Strike)} $$

A word on the PV(Strike)

For some candidates, it may be easier to understand the payoff concept without considering the strike’s present value (PV). This approach is acceptable for learning purposes. However, it is essential to note that the PV of the strike is included to account for the fact that acquiring a stock position requires an upfront payment. Put-call parity assumes that an investor can create positions without using their own money. Therefore, the PV of the strike indicates that the investor would need to borrow the amount required to purchase the stock and repay it with interest to maintain parity.

Conversely, investors who short a stock receive money from the sale. The sale proceeds are typically more than the cost of the purchased options. It is rational for investors to invest this money instead of leaving it idle. In the put-call parity equation, the term PV(strike) represents the present value of these funds. It signifies the potential earnings and interest that can be earned by investing the proceeds at a risk-free rate. Including this term is crucial for accuracy since it considers the time value of money and the opportunity cost of not investing the proceeds. Neglecting this term may result in slightly inaccurate answers that fail to account for the additional earnings or interest paid at the risk-free rate. 

Question

By ignoring the PV(Strike), an investor who buys a call option and sells a put option on the same underlying stock has most likely created?

1. A synthetic short.
2. A synthetic long.
3. A long stock position.

Solution

The correct answer is B.

When an investor purchases a call option, it provides the opportunity for potential gains above the strike price. On the other hand, selling a put option leads to losses below the strike price. Buying a call and selling a put creates a payoff profile similar to owning the underlying stock without actually owning it. Consequently, this position is known as a synthetic long position. It’s worth noting that adjustments for the time value of money account for the borrowing and lending related to the strike price. It’s important to mention that the investor benefits from price appreciation. However, unlike someone with equivalent shares, they won’t be entitled to collect dividends or vote proxies.

A is incorrect. A synthetic short involves selling an asset (like a stock) the investor does not own to repurchase at a lower price. In this case, investors have purchased call options to buy the stock at a specified strike price. This action doesn’t resemble a synthetic short, as the investor can buy the stock, not sell it.

C is incorrect. Long stock positions mean the investor owns the stock outright. Investors bought call options and sold put options in this scenario. Instead of owning the stock, they have the right to purchase it at a specific price (call option) or the obligation to purchase it at a specific price (put option). Thus, it does not represent an extended position. 

Derivatives and Risk Management: Learning Module 1: Options Strategies; Los 1(a) Demonstrate how an asset’s returns may be replicated by using options