Approaches to Asset Allocation and Statistical Characteristics and Challenges

Modeling risks and returns for alternative investments is challenging due to two key factors:

1. Stale Valuations: Private alternative investments often rely on appraisals for valuations, which can be outdated or artificially smoothed. This leads to underestimated fundamental risks when calculating volatility and other risk measures.
2. Non-Normal Distributions: Publicly traded asset classes can exhibit non-normal return distributions, but alternative investments are even more prone to skewness and fat tails (excess kurtosis). Factors like leverage, sensitivity to liquidity changes, and performance fee asymmetry contribute to this complexity, making statistical modeling less straightforward.

Skewness

Excess Kurtosis

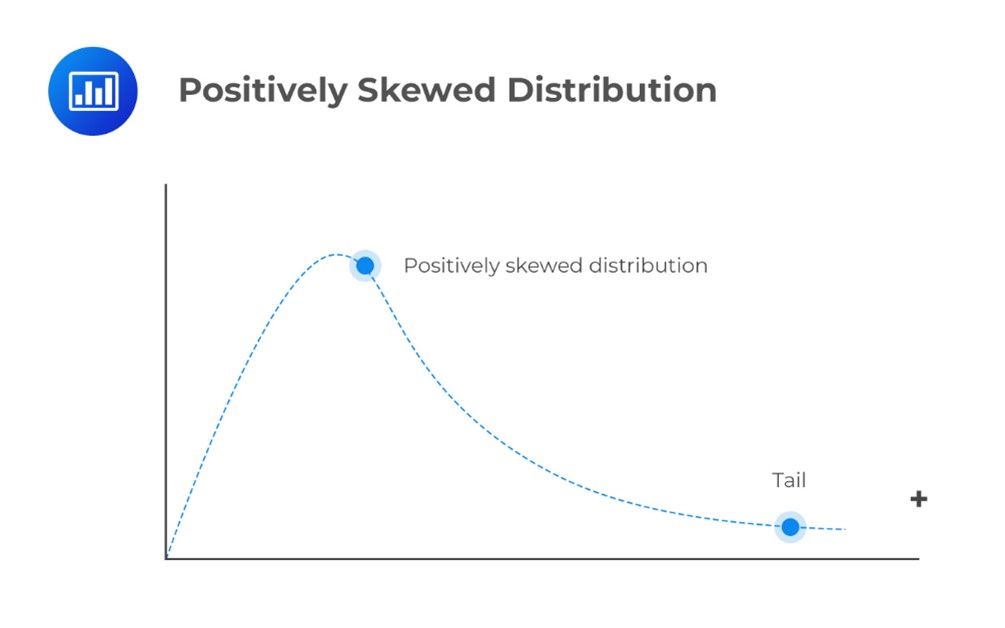
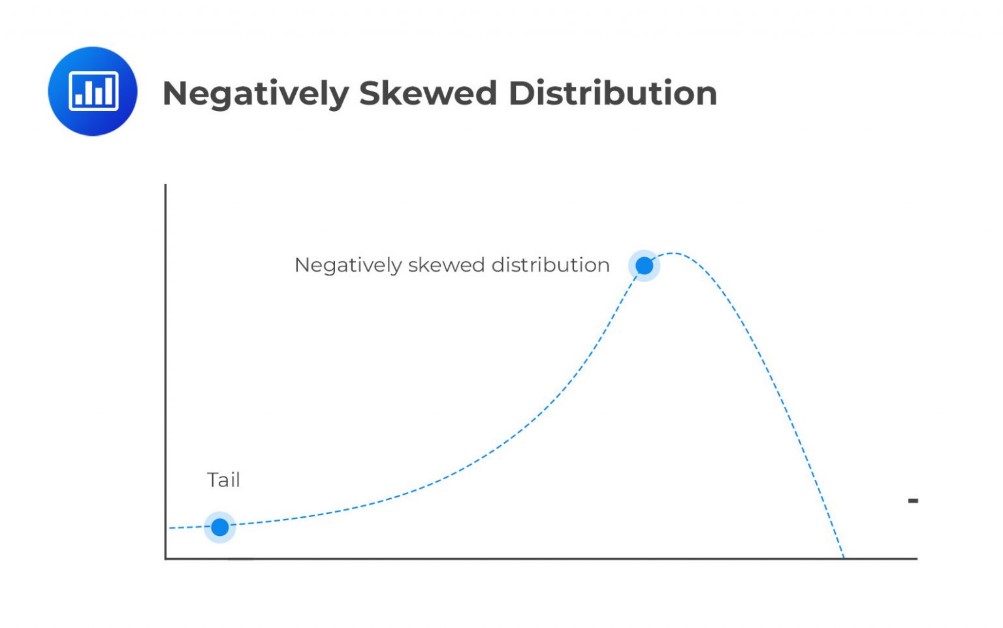
Candidates should understand that skewness and kurtosis in alternative assets make them behave differently from traditional assets. Modern Portfolio Theory (MPT) relies on normally distributed asset behavior, which is straightforward because all normal distributions look the same. But when skewness and kurtosis are introduced, it means the probabilities no longer fit this standard mold.

Asset allocators employ various approaches to address these challenges:

Monte Carlo Simulation

Monte Carlo Simulation involves generating numerous portfolio scenarios simulating different future outcomes. It’s like a statistical crystal ball. Parameters can be adjusted to match specific portfolio needs, and it doesn’t rely on the assumption of normally distributed returns.

Optimization Techniques

Mean-variance optimization (MVO) can sometimes over-allocate alternative assets because it underestimates risk due to infrequent pricing and the assumption of normal returns. To correct this bias, practitioners set allocation limits. Approaches like mean-CVaR optimization consider downside risk and skewness, providing a more accurate asset allocation.

Risk factor-based Approaches

These approaches assign expected returns, risk, and correlations to different factors impacting asset returns, akin to Fama-French factor models. However, risk factors can vary depending on the investor, and correlations can change in dynamic market conditions. Some factors remain stable, while others fluctuate.

These methods help navigate the challenges of alternative asset allocation by considering non-normal asset behaviors and optimizing portfolios accordingly.

Question

Which of the following is least likely a common statistical impediment to performing traditional MVO on a portfolio of alternative investments:

1. A bell curve.
2. Excess kurtosis.
3. Skewness.

Solution

The correct answer is A.

MVO is complex due to the diverse statistical distributions of returns seen in alternative investments. In modeling, we prefer a bell curve, representing a normal distribution, due to its known properties and probabilities.

B is incorrect. Kurtosis relates to the “height of the distribution.” In a normal distribution, most outcomes cluster around the center. But kurtosis means returns can concentrate elsewhere, more or less than expected.

These features add complexity to analyzing a portfolio of alternative investments.

C is incorrect. Skewness means the distribution is “off-centered,” like the Leaning Tower of Pisa. It’s an unhelpful property in statistical modeling.

Portfolio Construction: Learning Module 3: Asset Allocation to Alternative Investments; Los 3(f) Discuss approaches to asset allocation to alternative investments