Key Properties of the Normal Distribution

A random variable is said to have the normal distribution (Gaussian curve) if its values make a smooth curve that assumes a “bell shape.” A normal variable has a mean “μ,” pronounced as “mu,” and a standard deviation “σ,” pronounced as “sigma.” All normal distributions have a distinguishable bell shape regardless of the mean, variance, and standard deviation.

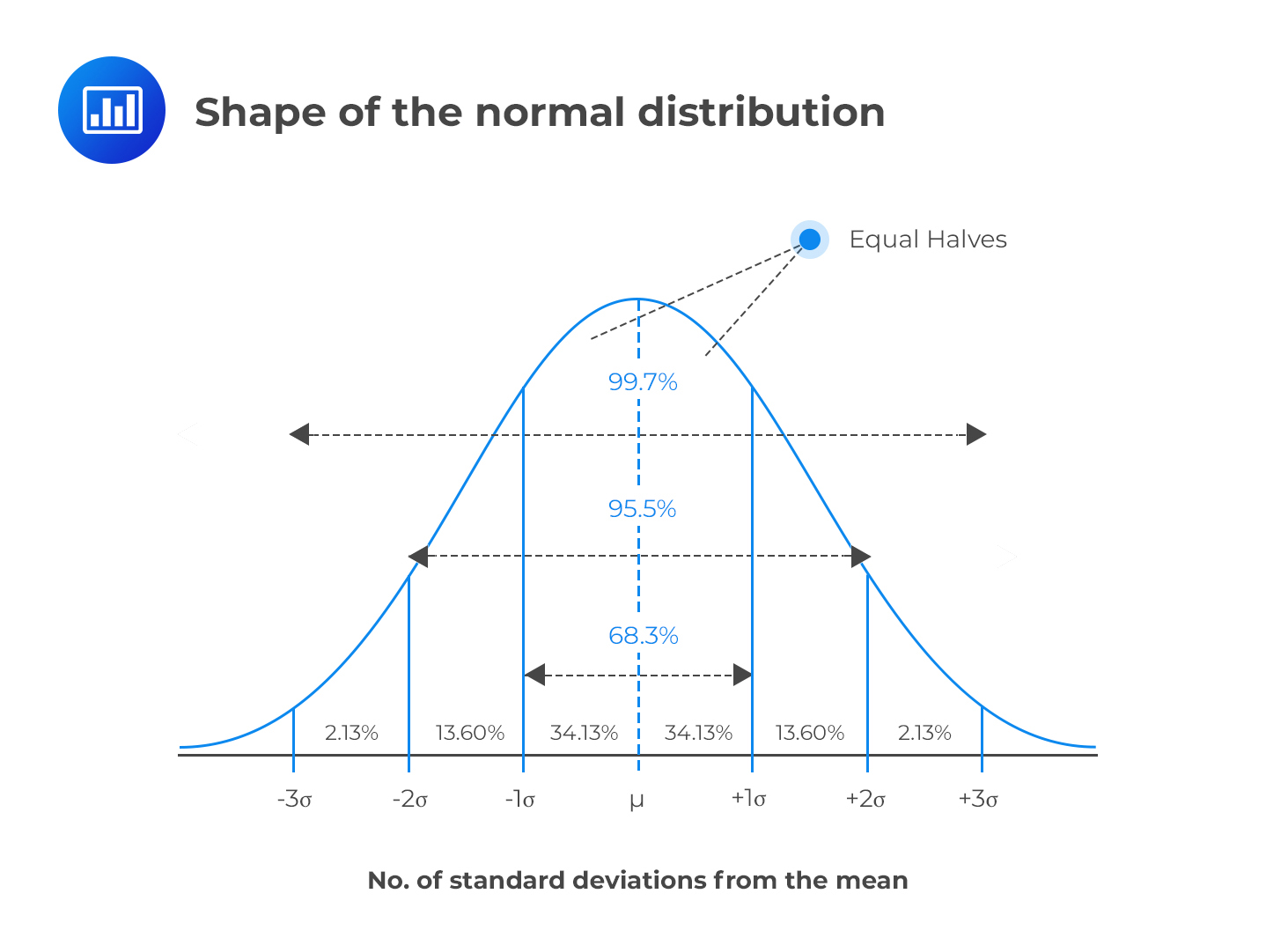
A normal distribution has certain properties that make it a useful tool in the world of finance.

1. Its shorthand notation is \(X \sim N(\mu , \sigma^2)\). This is read as “the random variable X has a normal distribution with mean μ and variance σ²”.
2. It has a symmetric shape: it can be cut into two halves that are mirror images of each other; as such, skewness = 0.
3. Kurtosis = 3. Note that kurtosis is a measure of flatness and excess kurtosis is measured relative to 3, the “normal kurtosis.”
4. The mean, mode, and median are all equal and lie directly in the middle of the distribution.
5. If \(X \sim N(\mu_x , \sigma^2_x)\) and \(Y\sim N(\mu_y , \sigma^2_y)\), then the two variables combined also have a normal distribution.
6. The standard deviation measures the distance from the mean to the point of inflection (the point where the curve changes an “upside-down-bowl” shape to a “right-side-up-bowl” shape).
7. Probabilities follow the empirical rule: first, about 68% of the total values lie within 1 standard deviation of the mean. In addition, 95% of the values lie within 2 standard deviations of the mean. Lastly, about 99.7% lie within 3 standard deviations of the mean.

Area Under the Normal Distribution Curve

To determine the probability that a random variable X lies between two points, ‘a’ and ‘b’:

$$ P\left( a < X < b \right) =\int _{ a }^{ b }{ f\left( x \right)dx } $$

The normal distribution is very important in statistical analysis, especially because of the central limit theorem. The theorem asserts that any distribution becomes normally distributed when the number of variables is sufficiently large. For instance, the binomial distribution tends to “change” into the normal distribution with mean nθ and variance nθ(1 – θ).

Reading 9 LOS 9i:

Explain the key properties of the normal distribution.