FRM Part I Practice Questions
FRM® Part I Study Materials and Question Bank
Get access to 2,000 exam-style FRM part 1 questions
Master the FRM Part I Exam with Confidence
The FRM Part I exam is tough—but with the right tools and strategy, you can walk into exam day fully prepared. AnalystPrep gives you everything you need to tackle even the trickiest concepts and walk away with confidence.
Why Choose AnalystPrep for Your FRM Part I Practice Questions?
At AnalystPrep, we understand the challenges of the FRM exam. That’s why our FRM Part I practice questions are meticulously designed to mirror the difficulty and style of the actual FRM exam part I.
Here’s how we set you up for success:
- Tailored Question Bank: Our exam-centered question bank focuses on the essential topics that make up the FRM curriculum, ensuring you grasp every critical concept.
- Regular Updates: We keep our content fresh by regularly updating the question bank to reflect the latest curriculum changes, so you’re always studying the most current material.
- Flexible Study Approaches: Whether you prefer tackling questions right after studying a topic or saving them for revision, our FRM question bank supports various study methods. Experiment and find what works best for you!
The FRM Part I Exam Structure
Exam Format
The FRM Part I exam is a comprehensive, computer-based test (CBT) that evaluates your understanding of risk management fundamentals. Here’s what to expect:
- 100 Multiple-Choice Questions: Each question is equally weighted, testing your knowledge across all topics.
- 4 Hours Total: You’ll have two sessions of two hours each to complete the exam.
- Practice-Oriented Assessment: The exam emphasizes practical application of risk management tools, techniques, and theories.
Exam Topics
The FRM Part I covers four main areas:
- Foundations of Risk Management
- Quantitative Analysis
- Financial Markets and Products
- Valuation and Risk Models
Focus on understanding the core concepts in each area to tackle any question that comes your way.
How Much Should You Study?
On average, candidates dedicate about 240 hours to prepare for the FRM Part I exam. However, study times can range from 100 to over 400 hours based on individual backgrounds and study habits.
Ask Yourself:
- Are you dedicating enough time to each topic?
- Have you balanced your study schedule to cover all exam areas?
Maximize Your Preparation with AnalystPrep
- Comprehensive Study Materials: Access our extensive FRM study material and video lessons to reinforce your learning.
- Mock Tests: Take our FRM mock tests to simulate the exam environment and track your progress.
- Detailed Explanations: Understand the reasoning behind each answer with our in-depth explanations, helping you learn from every mistake.
Ready to Ace the FRM Part I Exam?
Start your journey with AnalystPrep’s FRM Part I practice questions today! Explore our FRM question bank, take advantage of our mock tests, and access the latest FRM study materials to ensure you’re fully prepared for exam day.
Register Now and take the first step towards becoming a Certified Financial Risk Manager!
Affordable FRM Course Fees
Worried about the FRM course fees? AnalystPrep offers competitive pricing without compromising on quality. Invest in your future with our cost-effective study solutions:
CHECK OUT OUR FRM STUDY PACKAGES HERE

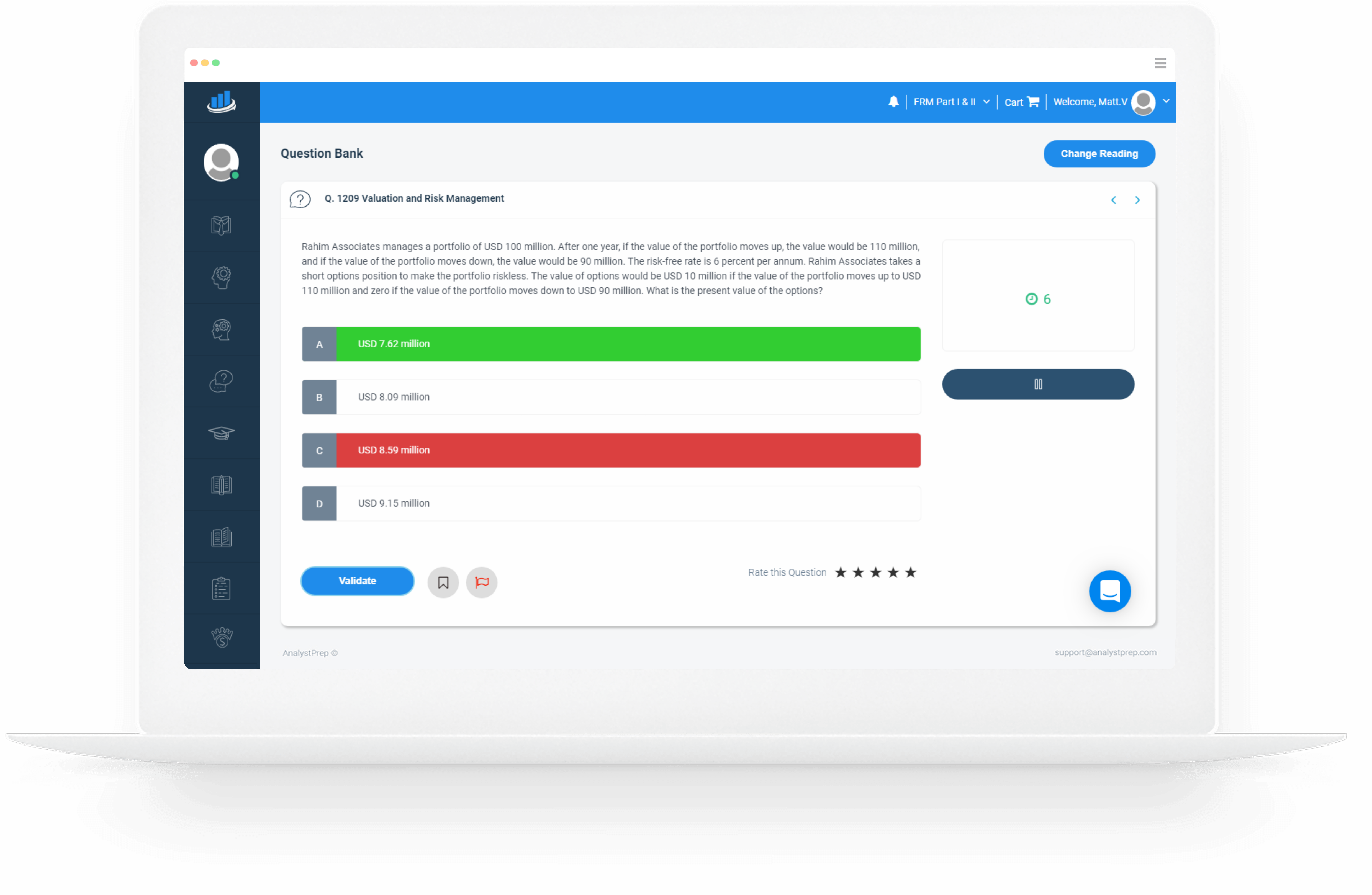
Quality and Quantity: Your Path to FRM Part I Success
When it comes to conquering the FRM Part I exam, the difference between success and failure often boils down to the quality of your preparation materials. With so many options available, how do you choose the best resources to ensure you pass? At AnalystPrep, we’ve got you covered!
Why Quality Matters More Than Quantity
FACT! The FRM exam is hard.
Each year, countless candidates hear both success and failure stories from their peers. To tip the scales in your favor, you need more than just a hefty stack of practice questions—you need high-quality, expertly curated materials that truly prepare you for the real thing.
While quality is paramount, quantity shouldn’t be overlooked. A robust question bank with a vast array of questions allows you to practice extensively, reinforcing your knowledge and boosting your confidence.
Our Approach to Balancing Quality and Quantity:
- Diverse Question Types: Our FRM mock tests include a variety of question types and scenarios, helping you adapt to different question formats and styles you’ll encounter on exam day.
- Simulated Exam Environment: Take our FRM mock exams to experience the computer-based testing (CBT) format. This simulation helps you manage your time effectively and get comfortable with the exam setup.
- Detailed Explanations: Each question in our question bank comes with in-depth explanations, allowing you to understand the reasoning behind each answer. This not only helps you learn from your mistakes but also reinforces your understanding of key concepts.
More Than Just Practice Questions
At AnalystPrep, we go the extra mile to ensure you have all the support you need to succeed.
Here’s What We Offer Beyond the Question Bank:
- Expert Instructors: Our FRM instructors are always on hand to provide additional tips and tricks, helping you navigate through challenging topics and optimize your study strategies.
- Interactive Learning: Engage with our video lessons and study notes that complement our question bank, offering a well-rounded preparation experience.
- Personalized Support: Whether you’re just starting your FRM preparation or revising for the final push, our team is here to support you every step of the way. From answering your queries to providing personalized study plans, we ensure you’re never alone in your journey.
Your FRM success story starts here!
Free FRM Part 1 Practice Questions
Question 1
Foundations of Risk Management
In the lead-up to the 2007/2009 financial crisis, Lehman Brothers had positioned itself as the leading institution in the mortgage-backed securities market. Which of the following best explains why the firm failed so spectacularly despite boasting huge amounts of capital?
A) The firm was highly leveraged, reducing its ability to absorb losses
B) A large number of the firm’s mortgage-backed securities were built upon sub-prime mortgage assets
C) The firm was considered too big to fail
D) A lack of confidence among investors which in turn led to a lack of funding
The correct answer is: A)
All of the above statements explain one aspect or the other about Lehman Brothers which eventually contributed to the firm’s failure. However, the firm’s main reason for failing can be traced down to the relationship between leverage and illiquidity. To fund its aggressive growth strategy, Lehman Brothers resorted to extreme leverage that far surpassed its capacity to repay. The firm took on huge amounts of short-term debt to fund long-term assets, exposing itself to serious liquidity problems. Too much debt meant that the firm could not absorb losses when the housing bubble burst.
Question 2
Financial Markets and Products
Alex Warner works as the senior derivatives manager at Warner Traders Limited. He’s currently managing a $120 million portfolio consisting of medium to large-cap U.S. equity. Warner wishes to lock up profit made during a recent rally and intends to use the S&P 500 futures with a multiplier of 250. At the moment, the S&P 500 and its futures are trading at USD 4,300 and USD 4,360, respectively, and Warner would like to hedge three-quarters of his market exposure over a three-month period. Warner’s portfolio has a correlation of 0.8 with the S&P 500 index futures, and the volatilities of the equity fund and the index futures are 0.55 and 0.50, respectively. To achieve his objective, Warner should:
A) Sell 73 futures contracts
B) Sell 61 futures contracts
C) Buy 61 futures contracts
D) Buy 73 futures contracts
The correct answer is: A)
The expression for the optimal hedge ratio, h, is as follows:
$$h=\rho\frac{\sigma_S}{\sigma_F}$$
where:
\(ρ\) = correlation between the portfolio and the index futures
\(\sigma_s\) = volatility of the portfolio
\(\sigma_F\) = volatility of the index futures
Thus,
$$h=0.8\times\frac{0.55}{0.5}=0.88$$
The optimal number of futures contracts needed, N, is determined as follows:
$$N=h\times\frac{Q_S}{Q_F}$$
where:
where:
\(h\) = hedge ratio
\(Q_S\) = Size of the position to be hedged
\(Q_F\) = Number of units of the asset underlying one futures contract,
Furthermore, note that only three-quarters of the total exposure needs to be hedged, i.e., 0.75 × USD 120 million = USD 90 million.
Thus,
$$N=0.88\times\frac{90,000,000}{4,360\times250}=72.67\approx73$$
Option B is incorrect. The optimal hedge ratio, h, is wrongly calculated as \(\rho\frac{\sigma_F}{\sigma_S}\)
C and D are incorrect. Since Warner has a long position in the underlying asset, he needs to take a short position (sell) to hedge the portfolio.
Question 3
Quantitative Analysis
A random sample of 50 FRM exam candidates was found to have an average I.Q. of 125. The standard deviation among candidates is known (approximately 20). Assuming that I.Q.s follow a normal distribution, carry out a statistical test (5% significance level) to determine whether the average I.Q. of FRM candidates is greater than 120. Compute the test statistic and give a conclusion.
A) Test statistic: 1.768; Reject \(H_0\)
B) Test statistic: 2.828; Reject \(H_0\)
C) Test statistic: 1.768; Fail to reject \(H_0\)
D) Test statistic: 1.0606; Fail to reject \(H_0\)
The correct answer is: A)
The first step: Formulate H0 and H1
$$\begin{align}H_0: μ &= 120\\ H1:μ & > 120\end{align}$$
Note that this is a one-sided test because H1 explores a change in one direction only
Under \(H_0\),
$$\frac{(\bar{X} – 120)}{(σ/\sqrt{n}) } \sim N(0,1)$$
Next, compute the test statistic:
$$= \frac{(125 – 120)}{(20/\sqrt{50})} = 1.768$$
Next, we know that \(P(Z > 1.6449) = 0.05\), which means our critical value is the upper 5% point of the normal distribution, i.e., 1.6449. Since 1.768 is greater than 1.6449, it lies in the rejection region. As such, we have sufficient evidence to reject H0 and conclude that the average I.Q. of FRM candidates is indeed greater than 120.
Alternatively, we could go the “p-value way.” The p-value is the smallest level of significance at which we can reject the null hypothesis
$$\text{p-value} = P(Z > 1.768) = 1 – P(Z < 1.768) = 1 – 0.96147 = 0.03853 \ \text{or}\ 3.853\%$$
This probability is less than 5%, meaning that there’s sufficient evidence against H0. This approach leads to the same conclusion – that the average I.Q. of FRM candidates is greater than 120.
Question 4
Valuation and Risk Models
A newly employed quantitative analyst at the risk management department of a systematically important bank (SIB) has developed a new risk measure (p) that could prove applicable to a wide range of risks at the bank. During validation and testing by an independent panel, she is asked to demonstrate that her measure is a coherent risk measure. She proceeds to formulate the following values:
- There are two portfolios – Y and Z
- y and z are state-contingent values of portfolios Y and Z, respectively
- P(y) and p(z) are the risk measures of the two portfolios
- b and c are constants where b > 0
- n is an amount in dollars
Which of the following equations effectively rules out p as a coherent risk measure?
A) P(y + z) ≤ p(y) + p(z)
B) P(by) ≤ bP(y)
C) P(y) ≥ p(z) if y ≤ z
D) $$\text{P(y + c) = p(z) – c}$$
The correct answer is: B)
Equation B demonstrates that the risk measure does not satisfy the homogeneity property.
The homogeneity property requires that \(\text{P(by) = bP(y)}\)
Interpretation: Increasing the size of a portfolio by a factor b should result in a proportionate scale in its risk measure. For example, if the value of b is 2, then the risk of the portfolio will be doubled.
Option A correctly demonstrates the subadditivity property.
Interpretation: If we add two portfolios together, the total risk can’t get any worse than adding the two risks separately.
Option C correctly demonstrates the monotonicity property.
Interpretation: If a portfolio has systematically lower values than another, it must have a greater risk in each state of the world. In other words, if a portfolio gives undesirables results than the others, then it must be riskier.
Question 5
Valuation and Risk Models
A trader holds options on a U.K stock that doesn’t pay dividends. The stock’s current price is USD 300, and the options will expire in a year. All of the options have a strike price of USD 280.
Which of the following is closest to the Black-Scholes values of these options?
Assumptions:
- Risk-free rate (continuously compounded) = 5%
- \(Nd_1\) = 0.7327
- \(Nd_2\) = 0.6164
A) Value of the American call option is USD 55.34 and that of the European put is USD 21.98
B) Value of the American call option is USD 21.98 and that of the European put is USD 0.00
C) Value of the American call option is USD 55.34 and that of the European put is USD 0.00
D) Value of the American call option is USD 21.98 and that of the European put is USD 55.34
The correct answer is: A)
For European options,
$$\text{Price}_{call}=P_0N\left(d_1\right)-Xe^{-rt}N\left(d_2\right)$$
$$\text{Price}_{put}=Xe^{-rt}\left(1-N\left(d_2\right)\right)-P_0\left(1-N\left(d_1\right)\right)$$
where:
\(P_0\) = spot price of the stock
\(X\) = options’ strike price
\(r\) = risk-free rate
\(t\) = time to expiration of the options
\(N(.)\) = cumulative normal distribution function Thus,
$$\text{Price}_{call}=300\times0.7317-280\times0.9512\times0.6164=55.34$$
$$\text{Price}_{put}=280\times0.9512\times0.3836-300\times0.2673=21.98$$
As seen from above, the value of each European call option is USD 55.34, while that of each put is USD 21.98. We also know that the value of an American option will never be less than that of an equivalent European option (same underlying, same expiration date, same strike), as it offers the same rights and more. We also know that the difference between American and European options is the early exercise feature of American-style options. But we also know that in the absence of dividends, an American call option has the same value as an equivalent European call option because there is no incentive to exercise early, and therefore the early exercise feature has no value. Thus, the only correct option is A.

FRM® Part 1
Our Learn + Practice package include study notes and video lessons for $499.
Combine FRM Part I and FRM Part II Learn + Practice for $799. This package includes unlimited ask-a-tutor questions and lifetime access with curriculum updates each year at no extra cost.
FRM Part 1 Practice Package
$
349
/ 12-month access
- Question Bank (Part 1)
- CBT Mock Exams (Part 1)
- Formula Sheet (Part 1)
- Performance Tracking Tools
FRM Part 1 Learn + Practice Package
$
499
/ 12-month access
- Question Bank (Part 1)
- CBT Mock Exams (Part 1)
- Formula Sheet (Part 1)
- Performance Tracking Tools
- Video Lessons (Part 1)
- Study Notes (Part 1)
FRM Unlimited Package (Part 1 and Part 2)
$
799
/ lifetime access
- Question Bank (Part 1 & Part 2 )
- CBT Mock Exams (Part 1 & Part 2 )
- Formula Sheets (Part 1 & Part 2)
- Performance Tracking Tools
- Video Lessons (Part 1 & Part 2 )
- Study Notes (Part 1 & Part 2 )













