GMAT® Focus Practice Questions
Get Access to 1,000 Exam-Style Questions
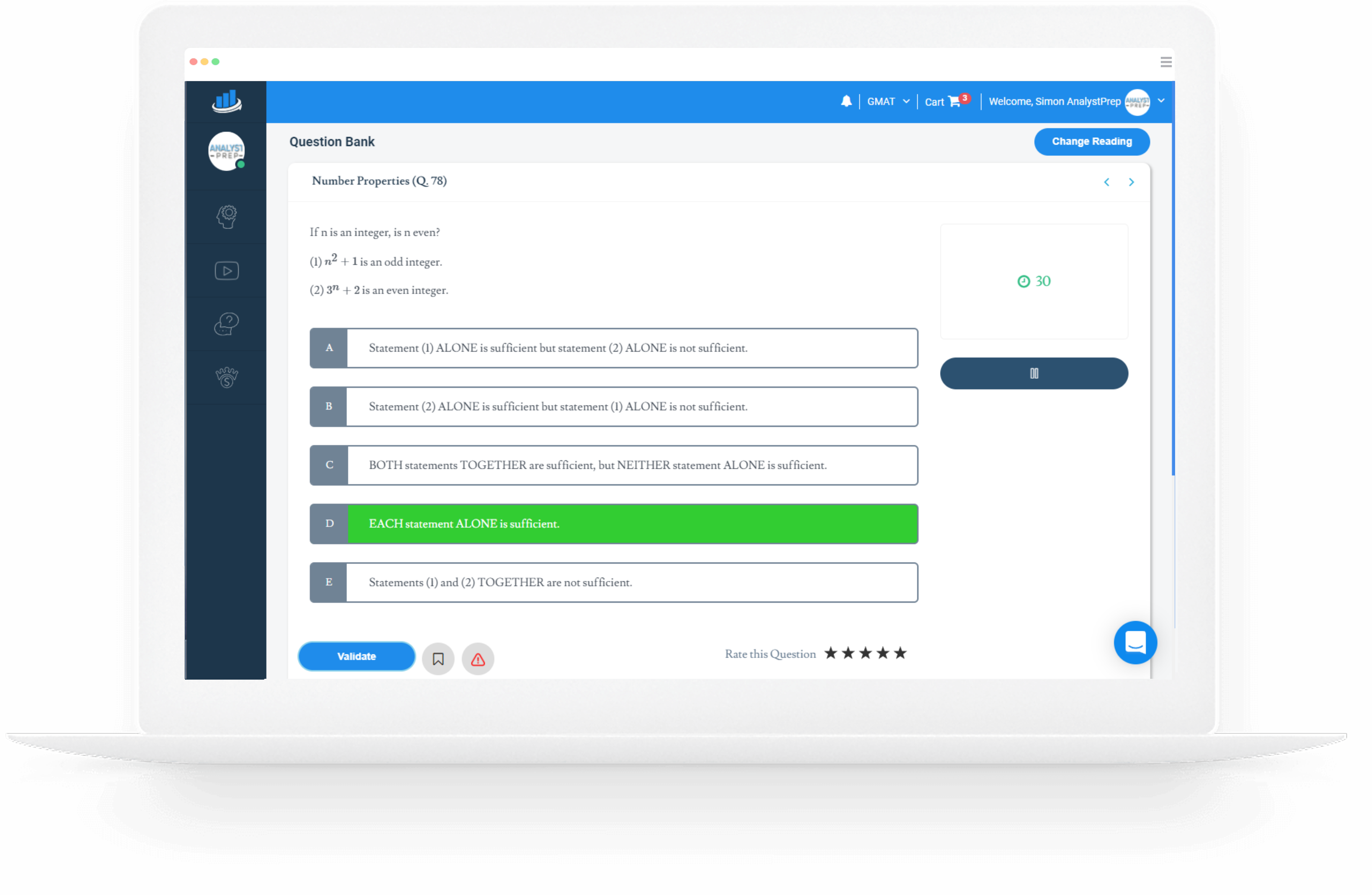
What Makes AnalystPrep’s Question Bank Unique?
Our question bank has been developed by certified professors with decades of experience teaching the GMAT® content. Our instructors know what it takes to pass. Each question is repeatedly validated to ensure you get the most out of the platform.
Preparing for the GMAT also means knowing what to expect during the actual test and familiarizing yourself with the types of questions you’re likely to encounter. At AnalystPrep, we adopt the exact question structure as the actual exam for quantitative, verbal, and even data insight questions. Most items consist of a stem (question, statement, and/or table) and five distracters – A, B, C, D, and E.
Our question bank is divided into specific topics—the same topics as for the video lessons—to help you attain an all-around understanding of the curriculum. Every question is accompanied by a detailed solution. In the event that you’d like some clarification on a specific concept, our instructors are reachable round the clock.
All our practice questions are adjusted regularly over time to be consistent with the current curriculum and difficulty level. Join the thousands of candidates who register yearly on our platform.
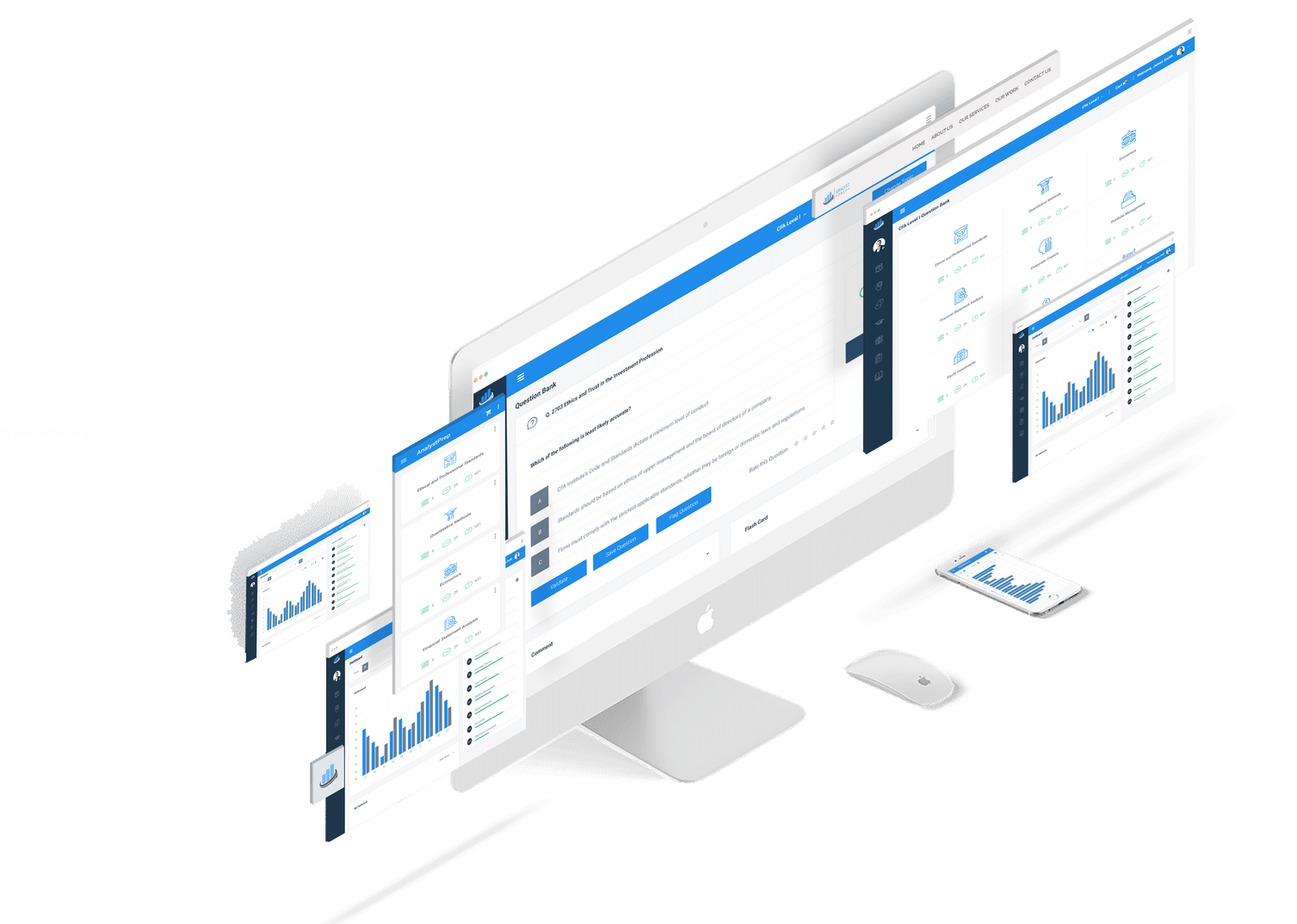
Performance Tracking Lets you Find your Strengths and Weaknesses
The GMAT Focus exam consists of many different topics covering a broad range of skills in a large volume of material. Testing your knowledge in each specific area by using the practice questions helps you understand where your strengths and weaknesses are. You can track your performance over time and monitor your progress with our performance tracking tool.
Performance tracking is part of our complete set of analytics tools that gives you invaluable insights into your performance, including the ability to compare your result with more than 50,000 users worldwide. Adjust your study plan according to the given results and improve your performance by revising the topics that you need to master. Efficient studying begins with knowing which topics you need to put more effort into so as to maximize your chances of passing.
Questions Answered by our Users
Satisfied Customers
Preparation Platform By Review Websites
Some Free Exam-style GMAT Focus Practice Questions offered by AnalystPrep
GMAT Quantitative Problems
Question 1
Division & Factoring
When integer y is divided by 2, is the remainder 1?
(1) \((-1)^{(y + 2)} = -1\)
(2) \(y\) is prime.
A) Statement (1) ALONE is sufficient but statement (2) ALONE is not sufficient.
B) Statement (2) ALONE is sufficient but statement (1) ALONE is not sufficient.
C) BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
D) EACH statement ALONE is sufficient.
E) Statements (1) and (2) TOGETHER are not sufficient.
The correct answer is: A)
(1) That \((–1)^{(y + 2)} = -1\), dictates that y + 2 must be odd and therefore y is odd since only an odd exponent can produce a value < 0. Furthermore, if an odd number is divided by 2, the remainder must be 1; SUFFICIENT.
(2) That y is prime does not definitively identify y as definitively even or odd, so the remainder could be either 0 or 1; NOT SUFFICIENT.
The correct answer is A; statement 1 alone is sufficient.
Question 2
Fractions and Decimals
Vivek bet \(\frac{1}{4}\) of his available gambling money on baseball, \(\frac{1}{6}\) on football, and \(\frac{1}{3}\) on basketball. If he didn’t bet $45 of his available gambling money, lost on the baseball and football bets, but won double on the basketball bet, which of the following represents Vivek’s current balance compared to his amount of gambling money before the three bets?
A) \(\frac{1}{4}\) more.
B) \(\frac{1}{6}\) more.
C) \(\frac{1}{12}\) more.
D) \(\frac{1}{12}\) less.
E) \(\frac{1}{6}\) less.
The correct answer is: D)
Set Vivek’s available gambling money as \(x\).
According to the problem, he wagered \(\frac{1}{4}x\), \(\frac{1}{6}x\) and \(\frac{1}{3}x\), or, using a common denominator of 12, \(\frac{9}{12}x\). This means that \(\frac{1}{4}\) of his money is $45 and \(x = $180\).
He lost \(\frac{1}{4}x + \frac{1}{6}x = $75\), but still has \( 2 × \frac{1}{3}x = $120\) from his basketball winnings + $45 he kept, so his total after the three bets is $165, which is $15 less than he had originally.
\(\frac {15}{180}=\frac {1}{12}\) that Vivek lost.
Question 3
Percentages (Data Insights)
In a vaccination line, 90 people have glasses and 80 people do not have glasses. 40% of the people with glasses have a pre-existing condition and 60% of the people without glasses have a pre-existing condition. If 75% of the people with glasses and pre-existing conditions receive a vaccination, and 50% of the people without glasses and with a pre-existing condition receive a vaccination, what percentage of all the people in line receive a vaccination?
A) 45
B) 40
C) 33
D) 30
E) 25
The correct answer is: D)
40% of the (90) people with glasses have a pre-existing condition. \(90 \times .4 = 36\) people with glasses and a pre-existing condition and 75% of this number receive a vaccination, so \(36 \times .75 = 27\) people with glasses and a pre-existing condition who receive a vaccination.
60% of the (80) people without glasses have pre-existing conditions. \(80 \times .6 = 48\) people without glasses and with pre-existing conditions. 50% of these people receive a vaccination, so \(48 \times .5 = 24\) people without glasses and with a pre-existing condition who receive a vaccination.
The total number of people with a pre-existing condition who receive a vaccination is 27 + 24 = 51. There are 90 + 80 people in line = 170 total, so the percentage of all people in line who receive a vaccination is \(\left(\frac {51}{170} \right) \times 100 = 30\%\).
Question 4
Exponents & Radicals (Data Insights)
The amount of bacteria in a culture after some \(t\) hours is given by the function \(f(t)=pe^{kt}\) where \(p\) is a constant. What is the number of bacteria after 8 hours?
(1) There were approximately 275 bacteria after 2 hours
(2) \(k=0.16\)
A) Statement (1) ALONE is sufficient but statement (2) ALONE is not sufficient.
B) Statement (2) ALONE is sufficient but statement (1) ALONE is not sufficient.
C) BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
D) EACH statement ALONE is sufficient.
E) Statements (1) and (2) TOGETHER are not sufficient.
The correct answer is: C)
(1) From the function, \(f(t)=pe^{kt}\), when \(t=2, f(t)=275\). The equation becomes \(pe^{2k}=275\). This is one equation in two unknowns, hence, we cannot solve it. More information is required; NOT SUFFICIENT.
(2) When \(k=0.16\), we have \(f(t)=pe^{0.16t}\). In this case, \(p\) is unknown, hence more information is required; NOT SUFFICIENT.
Considering the two cases, we have \(pe^{2k}=275\) and \(f(t)=pe^{0.16t}\) which reduced to \(pe^{2(0.16)}=275\). We solve the equation
$$\begin{align*} pe^{2(0.16)} &=275\\
pe^{3.2} &=275\\
\ln \left(pe^{3.2}\right) &=\ln 275\\
\ln p + 3.2 &=\ln 275\\
\ln p &=\ln 275 – 3.2 = 2.41677\\
p& =e^{2.41677}=11.21\end{align*}$$.
We now solve the equation
When \(t=8\), we have
$$f(8)=11.21e^{0.16(8)} =11.21e^{1.28}=40.32$$
The correct answer is C; BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
Question 5
Geometry (Data Insights)
A cylindrical layer cake has two vanilla layers surrounding a marzipan layer, represented by the shaded region, as shown below. Is the cake split into three layers of equal volume?
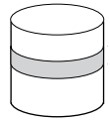
(1) The height of the marzipan layer is equal to the radius of the whole cake.
(2) The volume of the entire cake is 81π cubic inches.
A) Statement (1) ALONE is sufficient but statement (2) ALONE is not sufficient.
B) Statement (2) ALONE is sufficient but statement (1) ALONE is not sufficient.
C) BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
D) EACH statement ALONE is sufficient.
E) Statements (1) and (2) TOGETHER are not sufficient.
The correct answer is: E)
Note that the volume for a cylinder can be found from the formula \(πr^2 × height\) where r = radius and the height is perpendicular to the radius.
(1) That the height for the marzipan layer is equal to the radius of the cake means that the volume of the marzipan layer can be found from the simplified formula \(πr^3\), but the total cake volume remains dependent on the actual height of the cake; NOT sufficient.
(2) Represent the information algebraically as \(πr^2 × height = 81\), but no information is provided about the height in relation to the radius; NOT sufficient.
(Together) The information together still does not relate the height to the radius.
For instance, the radius could be 3 and the ratio of the marzipan layer to the others could be equal as each would have a volume of 27π.
Alternatively, the radius could = 1 and the marzipan layer would be significantly smaller than the other two layers.
The correct answer is E; both statements together are still not sufficient.
Question 6
Inequalities
At Rounders Grocer, orange slices cost $2 a pound, pineapple chunks cost $3 a pound, and cut watermelon cost $5 a pound. If Sally buys enough of these three fruits from Rounders to make five pounds of fruit salad, and at least one pound of each, which fruit did she buy the most of by weight?
(1) Sally spent less than $5 on orange slices.
(2) Sally spent more than $18 on her fruit salad.
A) Statement (1) ALONE is sufficient but statement (2) ALONE is not sufficient.
B) Statement (2) ALONE is sufficient but statement (1) ALONE is not sufficient.
C) BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
D) BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
E) Statements (1) and (2) TOGETHER are not sufficient.
The correct answer is: B)
First note that Sally must buy at least one pound of each of the fruits, which would result in a sum fixed cost of $10.
(1) If Sally spent less than $5 on orange slices, then she spent must have spent more on one of the other two fruits, but it is unclear which; NOT sufficient
(2) If Sally spent more than $18 then she must spend more than $8 on the two-variable pounds of fruit, which could only be accomplished by buying two more pounds of cut watermelon; Sufficient
The correct answer is B; statement 2 alone is sufficient.
Question 7
Reading Comprehension (Verbal Reasoning)
If King Tut were around today, could he send a text in Egyptian hieroglyphics? Yes, with the right font and keyboard. That’s because the writing system of the pharaohs has already been included in the Unicode Standard, meaning that a character like the Eye of Horus has a code point, 13080, that will render the same way on a tablet in Cairo and a smartphone in Beijing. Because Mayan hieroglyphs have yet to be encoded, the ancient Mayan emperor K’inich Janaab’ Pakal would have to stick to emoji—but that’s about to change.
Unicode is the international encoding standard that makes it possible for users to read, write, and search in a wide range of written languages on all manner of devices without technical miscommunication. Made up of a mix of academics, stakeholders, and interested volunteers, the Unicode Consortium has encoded 139 of the writing systems, technically known as scripts, ever to have existed. Given that alphabets like Cyrillic, Arabic, and Devanagari serve more than 60 languages each and that 500 languages use the Latin alphabet, Unicode makes electronic communication possible in almost a thousand languages. But there are more than a hundred writing systems to go.
In June 2017, the Unicode Consortium rolled out its tenth version in 26 years, which included four scripts as well as the Bitcoin sign and 56 new emoji. The scripts introduced this year include Nüshu, a writing system that was developed by women in the Hunan Province of nineteenth-century China as a workaround when they were denied formal education. Also newly available is Zanabazar Square, created by a Mongolian monk in the seventeenth century to write spiritual texts in Mongolian, Tibetan, and Sanskrit. Crucial as these steps toward cultural empowerment may be, it is the textable faces, socks, mermen, and the like that have brought this global standard into the limelight.
As Michael Erard, writer-in-residence at the Max Planck Institute for Psycholinguistics, recently pointed out in the New York Times, the incorporation of emoji into Unicode is a double-edged sword for those interested in preserving ancient and minority languages. While, on the one hand, emoji eat up the time of the already strapped volunteers who could be working on other scripts, customer demand for emoji also incentivizes voting members of the Unicode Consortium like Microsoft, Apple, and Google’s Alphabet to update their systems with each new version that Unicode releases.
What function does the phrase: “…a character like The Eye of Horus has a code point, 13080…” serve in the passage?
A) It provides an example of Unicode’s standardization across languages, countries, and platforms.
B) It indicates that an analogous Mayan hieroglyph would have the same code point.
C) It describes the order in which new languages are incorporated into the Unicode standard.
D) It describes the manner in which a particular character might be encoded into Unicode.
E) It provides an indication of the size of the Unicode character set.
The correct answer is: A)
A. Yes. This answer choice is stated explicitly in the first paragraph.
B. No. This answer choice is unsupported by the passage since no evidence is provided that analogous characters would have the same code point.
C. No. This answer choice is unsupported by the passage since no information is provided concerning the order in which new languages are incorporated.
D. No. This answer choice is unsupported by the passage since no information is provided concerning the manner in which languages are encoded.
E. No. This answer choice is unsupported by the passage since no information is provided concerning the size of the character set.
GMAT® Focus Prep Packages
GMAT Focus Complete Course
$
39
/ month
- Conceptual Video Lessons
- Quantitative Practice Problems
- Verbal Practice Problems
- Data Insight Practice Problems
- Customizable Quizzes
- Performance Tracking Tools





